nmsurobert
- 288
- 36
When looking at a diffraction pattern made by two slits, and trying to calculate wavelength, what do we count as fringes? For example, in this picture are there five total fringes or, like, 25?
I've been trying to set up a lab for my high school physics 2 class and the math says that every bright point should be considered a fringe, but I am having a hard time finding anything in writing that confirms what my math is telling me. Thanks!
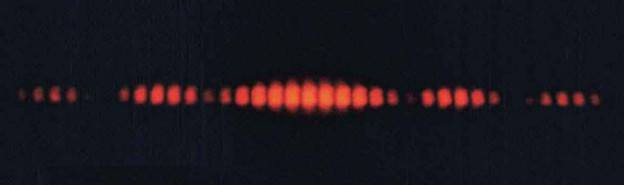
I've been trying to set up a lab for my high school physics 2 class and the math says that every bright point should be considered a fringe, but I am having a hard time finding anything in writing that confirms what my math is telling me. Thanks!