Obtaining a variable value from a 5-th degree polynomial in the tangent form

[baby_1]

TL;DR Summary

The integral of a 5-th polynomial in the tangent form

Hello,

Please see this part of the article.

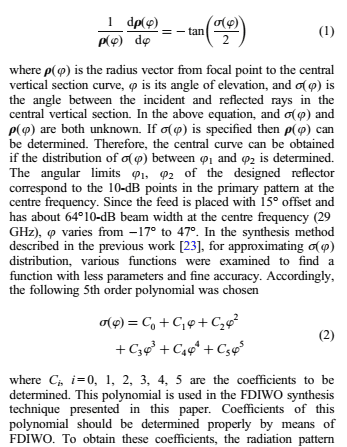
I need to obtain the $\rho (\phi)$ value after obtaining the c0 to c5 constants of the $\sigma (\phi)$. But as you can see after finding the coefficients, solving Eq.(1) could be a demanding job(I wasn't able to calculate the integral of Eq(1) and obtain the $\rho (\phi)$ ). I need to know how to find $\rho (\phi)$ values for different $\phi$ values.

$$\frac{1}{\rho (\phi )}\frac{d\rho (\phi)}{d\phi}= \\
tan(\frac{c_{0}+c_{1}\phi+c_{2}\phi^2+c_{3}\phi^3+c_{4}\phi^4+c_{5}\phi^5}{2})=>
\\ln(\rho (\phi ))=\int (tan(\frac{c_{0}+c_{1}\phi+c_{2}\phi^2+c_{3}\phi^3+c_{4}\phi^4+c_{5}\phi^5}{2})d\phi)$$

Or, I follow the wrong way.

The writers implied that after finding the $\sigma (\phi)$ the $\rho (\phi)$ could find easily.

 

[renormalize]

The writers implied that after finding the $\sigma (\phi)$ the $\rho (\phi)$ could find easily.

Your derived equation looks correct, provided you add the appropriate limits of integration. But I'm not convinced that the authors' "find easily" implication refers to performing the integration analytically in closed form. Instead, they may intend the integral to be performed numerically, which looks to be pretty straight forward once you have the values of the six C coefficients. The C's are apparently found from something called the "FDIWO synthesis technique". Is FDIWO a numerical technique involving something like FDTD or finite-elements? If so, that would suggest also using a numerical approach to evaluate your integral.

 

[baby_1]

I appreciate your help.
Yes, I don't understand how they obtain $\rho (\phi)$ for different values of $\phi$ -because this equation should be solved for many values of $\phi$.

No, the FDIWO is the acronym of frequency-dependent IWO- an optimization algorithm to find the C0 to Cn values-.

 

[renormalize]

Yes, I don't understand they obtain $\rho (\phi)$ for different values of $\phi$ -because this equation should be solved for many values of $\phi$.

With a computer you can perform this integration repeatedly and rapidly to generate a numerical table of $\rho (\phi)$ values.

No, the FDIWO is the acronym of frequency-dependent IWO- an optimization algorithm to find the C0 to Cn values-.

But my question was how this optimization is performed: analytically or numerically? Either way, once you have the C's, use repeated numerical integration to get your table of values.

 

[baby_1]

Thank you very much for your response. Yes, the optimization is performed numerically. So it means I should use the numerical form of the above equation instead of the analytical form.