- 2,163
- 191
We can write the Newtonian metric in the form of
$$ds^2 = -(1 - 2M/r)dt^2 + (1+2M/r)[dr^2 + r^2d\Omega^2]$$
In order to obtain the orbit equation I have written the constant of motion,
$$e = (1 - 2M/r)(\frac{dt}{d\tau})$$
and
$$l = r^2sin^2(\theta)(\frac{d\phi}{d\tau})$$
I can divide the metric to $$d \tau^2$$ and write
$$(\frac{ds}{d\tau})^2 = -(1 - 2M/r)(\frac{dt}{d\tau})^2 + (1+2M/r)[(\frac{dr}{d\tau})^2 + r^2((\frac{d\theta}{d\tau})^2 + r^2sin^2(\theta)(\frac{d\phi}{d\tau})^2]$$
by using ##e## and ##l##, and from the initial conditions we know that ##(\frac{d\theta}{d\tau}) = 0## for ##\theta = \pi /2 ##
$$-1 = -(1-2M/r)^{-1}e^2 + (1+2M/r)[(u^r)^2 + l^2/r^2]$$
where ##(u^r)^2 = (\frac{dr}{d\tau})^2##
I want to format this equation in such a way that I would obtain similar to
$$ e^2 = \frac{1}{2} (u^r)^2 + \text{terms}$$
where some terms will give the effective potential energy.
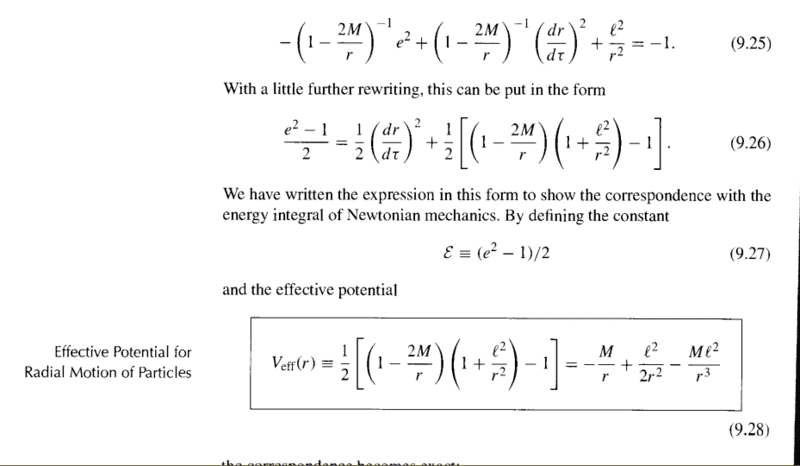
Here is a version of it done for the Schwarzschild Metric
$$ds^2 = -(1 - 2M/r)dt^2 + (1+2M/r)[dr^2 + r^2d\Omega^2]$$
In order to obtain the orbit equation I have written the constant of motion,
$$e = (1 - 2M/r)(\frac{dt}{d\tau})$$
and
$$l = r^2sin^2(\theta)(\frac{d\phi}{d\tau})$$
I can divide the metric to $$d \tau^2$$ and write
$$(\frac{ds}{d\tau})^2 = -(1 - 2M/r)(\frac{dt}{d\tau})^2 + (1+2M/r)[(\frac{dr}{d\tau})^2 + r^2((\frac{d\theta}{d\tau})^2 + r^2sin^2(\theta)(\frac{d\phi}{d\tau})^2]$$
by using ##e## and ##l##, and from the initial conditions we know that ##(\frac{d\theta}{d\tau}) = 0## for ##\theta = \pi /2 ##
$$-1 = -(1-2M/r)^{-1}e^2 + (1+2M/r)[(u^r)^2 + l^2/r^2]$$
where ##(u^r)^2 = (\frac{dr}{d\tau})^2##
I want to format this equation in such a way that I would obtain similar to
$$ e^2 = \frac{1}{2} (u^r)^2 + \text{terms}$$
where some terms will give the effective potential energy.
Here is a version of it done for the Schwarzschild Metric
Last edited: