Optimal thin absorber of electromagnetic energy

[Leo2024]

Hi, I am a material engineer and have a question about a formula derivation relative to microwave absorption. I really cannot figure it out after days of trying. This should be simple for a specialist.

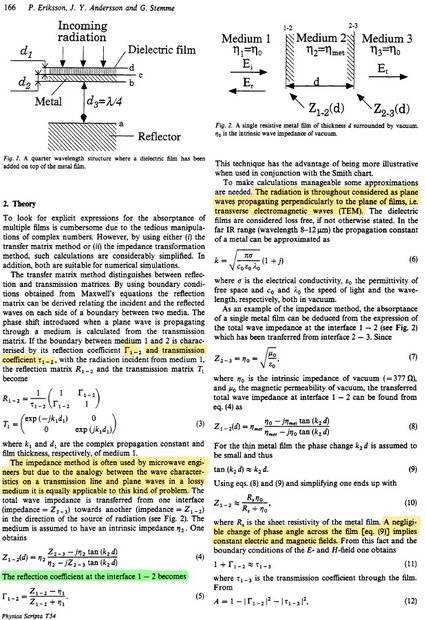
In this attached paper, how could one derive Eq(10) based on Eq (8) and (9)? Is k_2 in Eq (8) a complex number?

Source: https://www.researchgate.net/profil...of-the-permeable-base-transistor.pdf#page=168

 

[berkeman]

Thread closed temporarily for Moderation...

 

[berkeman]

Thread is reopened provisionally...

 

[Tom.G]

I read eq. (9), and its surrounding text, as saying that the complex part is so small that that it can be ignored - leading to eq. (10) being real (with the complex part negligible).

Caveat: Not an expert in the field, just my interpretation of the text and equations.

Cheers,
Tom

 

[renormalize]

In this attached paper, how could one derive Eq(10) based on Eq (8) and (9)? Is k_2 in Eq (8) a complex number?

You can rewrite eq.(8) of the paper in the form:$$Z_{1-2}\left(d\right)=\eta_{0}\left(\frac{\eta_{\mathrm{met}}}{\eta_{0}}\right)\left(\frac{1-j\left(\frac{\eta_{\mathrm{met}}}{\eta_{0}}\right)\tan\left(k_{2}d\right)}{\left(\frac{\eta_{\mathrm{met}}}{\eta_{0}}\right)-j\tan\left(k_{2}d\right)}\right)\tag{1}$$You already know from eq.(9) that $\left|k_{2}d\right|\ll1$, but it's also true that $\left|\eta_{\mathrm{met}}/\eta_{0}\right|\ll1$ because the impedance of a good-conducting metal is very small. So we can expand (1) to lowest order in the two small quantities to get:$$Z_{1-2}\left(d\right)\approx\frac{\eta_{0}\left(\frac{\eta_{\mathrm{met}}}{\eta_{0}}\right)}{\left(\frac{\eta_{\mathrm{met}}}{\eta_{0}}\right)-jk_{2}d}=\frac{\eta_{0}\,\eta_{\mathrm{met}}}{\eta_{\mathrm{met}}-j\eta_{0}\,k_{2}d}\tag{2}$$Now rewrite eq.(6) and the definition of impedance, in terms of the metal's conductivity $\sigma_{\mathrm{met}}$ and skin-depth $\delta_{s}\equiv\sqrt{\frac{2}{\omega\mu\sigma_{\mathrm{met}}}}$:$$k_{2}=\frac{1+j}{\delta_{s}},\;\eta_{\mathrm{met}}\equiv\frac{\omega\mu}{k_{2}}=\frac{1-j}{\sigma_{\mathrm{met}}\,\delta_{s}}\tag{3a,b}$$Finally, insert this into (2) to yield:$$Z_{1-2}\left(d\right)\approx\frac{\eta_{0}}{1+\eta_{0}\,\sigma_{\mathrm{met}}d}=\frac{R_{S}\,\eta_{0}}{R_{S}+\eta_{0}}\tag{4}$$where $R_{S}\equiv1/\left(\sigma_{\mathrm{met}}d\right)$.

(Edited to include absolute-value signs in the inequalities.)