- #1
Master1022
- 611
- 117
- Homework Statement
- A laboratory owns a group of special microscopes and it has been agreed that a replacement policy should be established. Using the data below and a discount rate of 10%, find the optimum replacement period and the average annual cost of ownership over this period. The purchase price when new should be taken as $18,000. For the purposes of calculation you should assume that the running costs are incurred at the end of each year.
- Relevant Equations
- Discount Factor
Hi,
I have a question about doing these types of optimum replacement problems.
Question: A laboratory owns a group of special microscopes and it has been agreed that a replacement policy should be established. Using the data below and a discount rate of 10%, find the optimum replacement period and the average annual cost of ownership over this period. The purchase price when new should be taken as $19,000. For the purposes of calculation you should assume that the running costs are incurred at the end of each year.
(we are provided the table in the data below)
[tex]
\begin{array}{|c|c|c|c|c|}
\hline & \text{Year 1} & \text{Year 2} & \text{Year 3} & \text{Year 4} \\
\hline \text{Running Cost} ($) & 3000 & 3600 & 3900 & 5100 \\
\hline \text{Value at end of year} ($) & 13000 & 10000 & 8500 & 7200 \\
\hline
\end{array} [/tex]
Attempt:
I do not really understand how to tackle this type of problem. Do we just need to find the year when it is more expensive to keep the car than to replace the car?
We can apply the discount rate as [itex] (1 + \frac{d}{100})^{-t} = (1.1)^{-t} [/itex]
I have discounted each of the numbers in the table using the above formula. I am not sure how to combine the numbers to come to a conclusion.
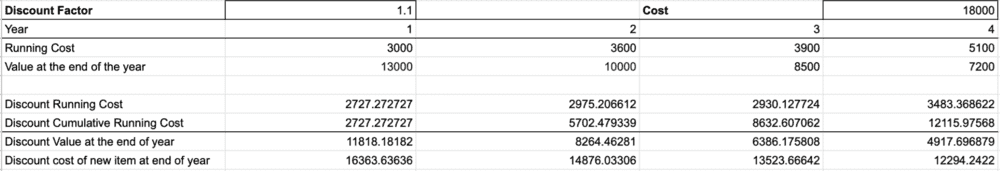
I think in general that we want to replace the item when it is more expensive to keep it than to replace it.
Any help would be greatly appreciated
I have a question about doing these types of optimum replacement problems.
Question: A laboratory owns a group of special microscopes and it has been agreed that a replacement policy should be established. Using the data below and a discount rate of 10%, find the optimum replacement period and the average annual cost of ownership over this period. The purchase price when new should be taken as $19,000. For the purposes of calculation you should assume that the running costs are incurred at the end of each year.
(we are provided the table in the data below)
[tex]
\begin{array}{|c|c|c|c|c|}
\hline & \text{Year 1} & \text{Year 2} & \text{Year 3} & \text{Year 4} \\
\hline \text{Running Cost} ($) & 3000 & 3600 & 3900 & 5100 \\
\hline \text{Value at end of year} ($) & 13000 & 10000 & 8500 & 7200 \\
\hline
\end{array} [/tex]
Attempt:
I do not really understand how to tackle this type of problem. Do we just need to find the year when it is more expensive to keep the car than to replace the car?
We can apply the discount rate as [itex] (1 + \frac{d}{100})^{-t} = (1.1)^{-t} [/itex]
I have discounted each of the numbers in the table using the above formula. I am not sure how to combine the numbers to come to a conclusion.
I think in general that we want to replace the item when it is more expensive to keep it than to replace it.
Any help would be greatly appreciated
Attachments
Last edited: