Oscillating dipoles, energy and brightness

[milkism]

Homework Statement

Brightness as function of time.

Relevant Equations

See solution.

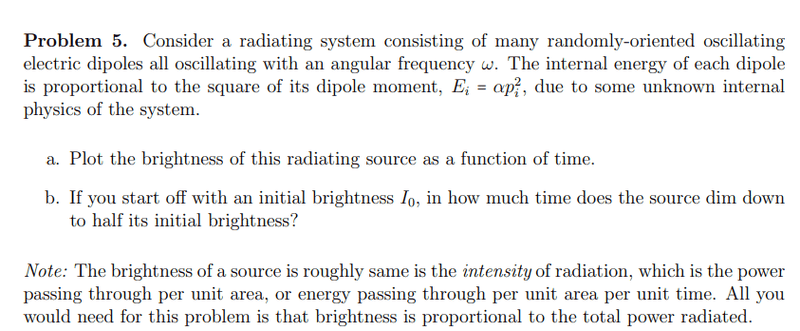
Problem:

Attempt at solution:
So "energy passing through per unit area per unit time" is equal to $$I = \frac{E_i}{A t}$$
So for a the graph will be in the form of $y=1/x$?
For b) do we have to solve the differential equation $$dI = \frac{E_i}{A dt}$$?

 

[TSny]

Attempt at solution:
So "energy passing through per unit area per unit time" is equal to $$I = \frac{E_i}{A t}$$

$E_i$ is not the energy that should appear in the above formula. The intensity of radiation $I$ at some point of space is the flux of radiation energy at that point. But $E_i$ is not radiation energy. $E_i$ is defined in the problem as the internal energy of the $i$th oscillator.

For this problem, use the hint that the brightness is proportional to the total power radiated by the oscillators.

Note that as a dipole radiates, the internal energy $E_i$ of the dipole is converted into radiation energy. Assume that the internal energy of the dipole is not being replenished. So, the internal energy of the dipole will decrease over time.

Try to discover the differential equation that describes the rate of loss of internal energy of a dipole. I believe you will need to know the formula for the power radiated by an oscillating electric dipole in terms of its angular frequency $\omega$ and its dipole moment $p$.