Oscillations in an LC circuit (Question from Irodov)

[Anubhav]

Homework Statement:

1. The problem statement, all variables and given/known data
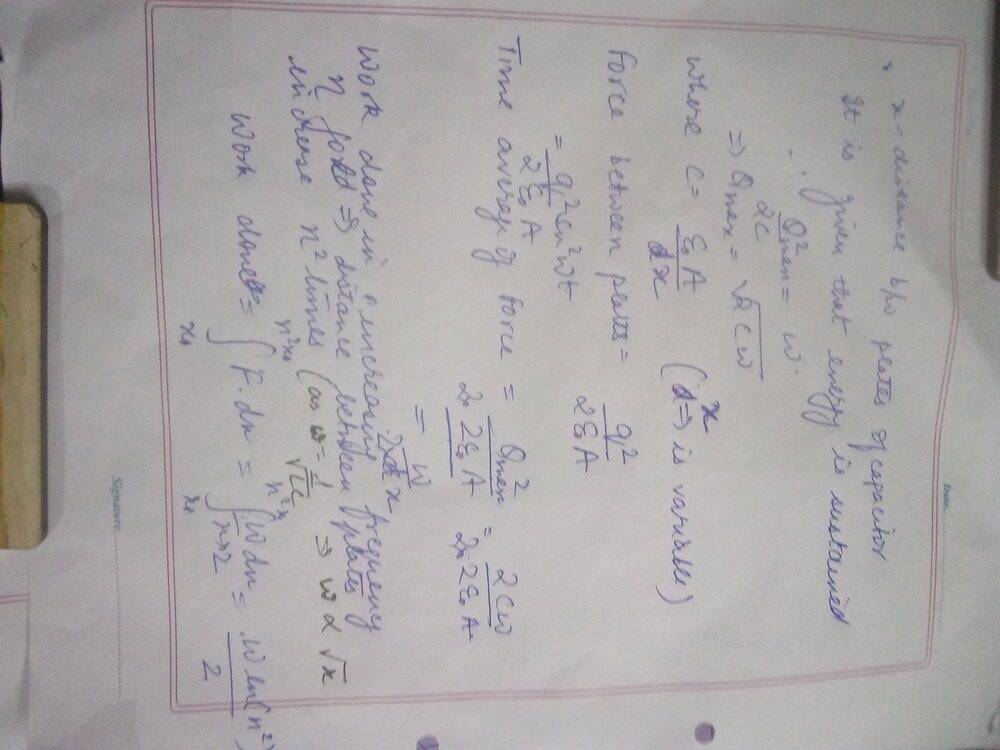
In an oscillating circuit consisting of of a parallel-plate capacitor and an inductance coil with negligible active resistance the oscillations with energy are sustained. The capacitor plates were slowly drawn apart to increase the scillation frequency -fold. What work was done in the process?

Relevant Equations:

Variables have meaning as usual
€=permitivity of vaccum
Energy in LC oscillations =W
Energy of charged capacitor=(Q^2)/2C
Force between plates of parallel plate capacitor=(Q^2)/2€A

https://www.physicsforums.com/attachments/282131

 

[BvU]

Figure is lost, your effort (attempt at solution) is missing ...

Please read
https://www.physicsforums.com/threads/homework-help-guidelines-for-students-and-helpers.686781/

$\\$

 

[Anubhav]

Sorry for inconvenience .I will type the solution

Figure is lost, your effort (attempt at solution) is missing ...

Please read
https://www.physicsforums.com/threads/homework-help-guidelines-for-students-and-helpers.686781/

$\\$

 

[BvU]

For those who want to read it without breaking their neck ...

I can also decipher another relevant equation ($\ \omega = \displaystyle {1\over\sqrt{LC}}\$)
So inceasing $\omega$ n times means changing $\ d \rightarrow n^2d\$.
Unclear to me what is conserved -- is $\ q\$ the same as $\ Q_{\text{max}}\$ ? and is that invariant ?
Then where does the mechanical work energy go ?

$\$

 

[Anubhav]

I'm sorry for inconvenience ..I shall delete this thread and reframe the entire Q properly again

 

[BvU]

Pinging @DaveE

Congratulations with the badge ! I'm sure there is no broom, and I'm certain they don't have cookies either (my clumsy way to distinguish you from @davenn)​

​

@Anubhav shouldn't apologize too much. Plenty effort demonstrated. I'm just filing away at his (her?) assumptions to test them. Can you handle this one in a more didactically responsible way ?

I ask myself: Would there be much difference between this case of changing $d$ and the DC case ?

$\$

 

[Anubhav]

Pinging @DaveE

Congratulations with the badge ! I'm sure there is no broom, and I'm certain they don't have cookies either (my clumsy way to distinguish you from @davenn)​

​

@Anubhav shouldn't apologize too much. Plenty effort demonstrated. I'm just filing away at his (her?) assumptions to test them. Can you handle this one in a more didactically responsible way ?

I ask myself: Would there be much difference between this case of changing $d$ and the DC case ?

$\$

Hello sir..Thanks for your I read your reply and gave it a thought again...
Also I have posted a typed image of Question as certain elements were missing in Q which I wrote
I am happy to inform you that after discussion with my friends I was able to solve it and got the right answer.
Actually I had assumed the Total energy of LC oscillations to be constant. Obviously that was wrong.
In my another attempt I wrote Change in energy of LC oscillations [i.e change in Qmax^2/(2C)] as function of integration of F(x).dx (where x is distance between plates)
Differentiation of this leads to a differential equation solving which gives Qmax as function of x..
Then change in energy of LC oscillations gives Total work done.
Ans comes out to be W(n-1)

 

[rude man]

Assumption: current does not change. (No dissipation, is like amperian currents in a permanent magnet).
So:
$V_C \rightarrow nV_C$
$V^2_C \rightarrow n^2V^2_C$
$C \rightarrow C/n^2$
So $1/2 ~CV_C^2$ is unchanged; inductance energy is unchanged; circuit energy is unchanged; work = 0.

Probably wrong somewhere.Probably assumption of no current change.