- #1
- 1,098
- 1,385
- Homework Statement
- Determine the period of small oscillations, given that the disc rolls without slipping
- Relevant Equations
- N/A
A disc of radius ##a## has a smaller disc of radius ##a/2## removed. The resulting object has mass ##m##:
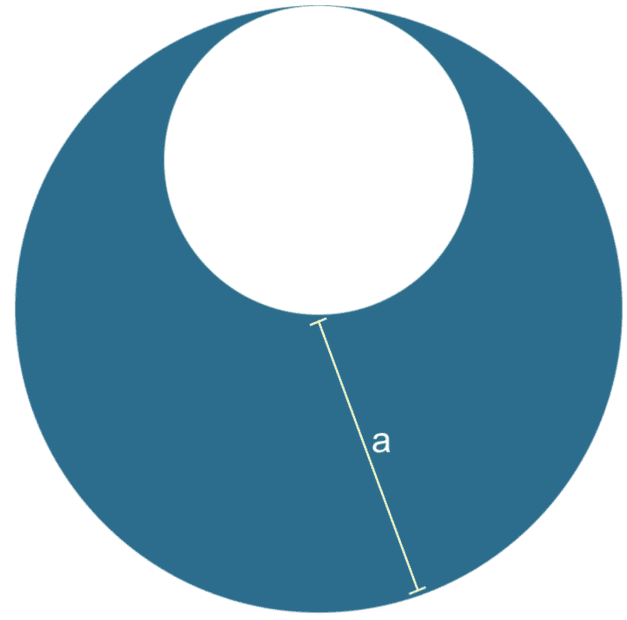The centre of mass ##G## is a distance ##h = \dfrac{\pi a^3 - \dfrac{3\pi a^3}{8} }{\dfrac{3\pi a^2}{4}} = \dfrac{5a}{6}## from the edge. The moment of inertia of the shape about the centre ##C## is\begin{align*}
I_C = \dfrac{1}{2}(\pi a^2 \rho)a^2 - \left(\dfrac{\pi a^2 \rho}{4} \right) \left( \dfrac{1}{2} \left(\dfrac{a}{2} \right)^2 + \left(\dfrac{a}{2} \right)^2 \right) = \dfrac{13\pi a^4 \rho}{32}
\end{align*}therefore the moment of inertia about ##G## is $$I_G = I_C + \dfrac{3\pi a^2 \rho}{4} \left(\dfrac{a}{6} \right)^2 = \dfrac{41 \pi a^4 \rho}{96}$$If the angle through which the disc has rotated is denoted ##\varphi##, then the centre of mass ##G## has velocity\begin{align*}
\mathbf{v}_G &= (a\dot{\varphi})\mathbf{\hat{x}} + \boldsymbol{\omega} \times \overrightarrow{CG} = \left(a\dot{\varphi} - \dfrac{a \dot{\varphi}}{6} \cos{\varphi} \right)\mathbf{\hat{x}} + \left(\dfrac{a\dot{\varphi}}{6} \sin{\varphi} \right)\mathbf{\hat{y}} \\
\implies v_G^2 &= \frac{a^2 \dot{\varphi}^2}{36}\left(37 - 12\cos{\varphi} \right)
\end{align*}Therefore the kinetic energy of the body is\begin{align*}
T = \dfrac{1}{2} m v_G^2 + \dfrac{1}{2}I_G \dot{\varphi}^2 = \pi a^4 \rho \dot{\varphi}^2 \left( \dfrac{1}{96}(37 - 12\cos{\varphi}) + \frac{41}{192} \right) = \pi a^4 \rho \dot{\varphi}^2 \left( \dfrac{115}{192} - \dfrac{1}{8} \cos{\varphi} \right)
\end{align*}Meanwhile, the height of the centre of mass ##G## when the body has rotated by ##\varphi## is\begin{align*}
y_G = a(1-\cos{\varphi}) + \dfrac{5a}{6} \cos{\varphi} = a(1- \dfrac{1}{6} \cos{\varphi})
\end{align*}therefore the potential energy is ##U = \dfrac{3\pi a^3 \rho g}{4}\left(1- \dfrac{1}{6} \cos{\varphi} \right)##. Overall I write the energy ##E## as\begin{align*}
\dfrac{1}{\pi a^3 \rho} E &= a \dot{\varphi}^2 \left( \dfrac{115}{192} - \dfrac{1}{8} \cos{\varphi} \right) + \dfrac{3g}{4}\left(1- \dfrac{1}{6} \cos{\varphi} \right) \\ \\
\implies \dot{E} &= 2a \dot{\varphi} \ddot{\varphi}\left( \dfrac{115}{192} - \dfrac{1}{8} \cos{\varphi} \right) + \dfrac{a\dot{\varphi}^3}{8} \sin{\varphi} + \dfrac{g \dot{\varphi}}{8} \sin{\varphi} \overset{!}{=} 0
\end{align*}The motion does not look simple harmonic, at least for finite ##\varphi##. To investigate we can put ##\cos{\varphi} = 1## and ##\sin{\varphi} = \varphi##, and I assume also ##\dot{\varphi}^2 = 0##, which gives\begin{align*}
\ddot{\varphi} + \dfrac{12g}{91a} \varphi = 0 \implies \Omega &= \sqrt{\dfrac{12 g}{91 a}} \\
T &= \pi \sqrt{\dfrac{91a}{3g}}
\end{align*}However in the solution manual they write ##T = \pi \sqrt{29a/g}##?
I_C = \dfrac{1}{2}(\pi a^2 \rho)a^2 - \left(\dfrac{\pi a^2 \rho}{4} \right) \left( \dfrac{1}{2} \left(\dfrac{a}{2} \right)^2 + \left(\dfrac{a}{2} \right)^2 \right) = \dfrac{13\pi a^4 \rho}{32}
\end{align*}therefore the moment of inertia about ##G## is $$I_G = I_C + \dfrac{3\pi a^2 \rho}{4} \left(\dfrac{a}{6} \right)^2 = \dfrac{41 \pi a^4 \rho}{96}$$If the angle through which the disc has rotated is denoted ##\varphi##, then the centre of mass ##G## has velocity\begin{align*}
\mathbf{v}_G &= (a\dot{\varphi})\mathbf{\hat{x}} + \boldsymbol{\omega} \times \overrightarrow{CG} = \left(a\dot{\varphi} - \dfrac{a \dot{\varphi}}{6} \cos{\varphi} \right)\mathbf{\hat{x}} + \left(\dfrac{a\dot{\varphi}}{6} \sin{\varphi} \right)\mathbf{\hat{y}} \\
\implies v_G^2 &= \frac{a^2 \dot{\varphi}^2}{36}\left(37 - 12\cos{\varphi} \right)
\end{align*}Therefore the kinetic energy of the body is\begin{align*}
T = \dfrac{1}{2} m v_G^2 + \dfrac{1}{2}I_G \dot{\varphi}^2 = \pi a^4 \rho \dot{\varphi}^2 \left( \dfrac{1}{96}(37 - 12\cos{\varphi}) + \frac{41}{192} \right) = \pi a^4 \rho \dot{\varphi}^2 \left( \dfrac{115}{192} - \dfrac{1}{8} \cos{\varphi} \right)
\end{align*}Meanwhile, the height of the centre of mass ##G## when the body has rotated by ##\varphi## is\begin{align*}
y_G = a(1-\cos{\varphi}) + \dfrac{5a}{6} \cos{\varphi} = a(1- \dfrac{1}{6} \cos{\varphi})
\end{align*}therefore the potential energy is ##U = \dfrac{3\pi a^3 \rho g}{4}\left(1- \dfrac{1}{6} \cos{\varphi} \right)##. Overall I write the energy ##E## as\begin{align*}
\dfrac{1}{\pi a^3 \rho} E &= a \dot{\varphi}^2 \left( \dfrac{115}{192} - \dfrac{1}{8} \cos{\varphi} \right) + \dfrac{3g}{4}\left(1- \dfrac{1}{6} \cos{\varphi} \right) \\ \\
\implies \dot{E} &= 2a \dot{\varphi} \ddot{\varphi}\left( \dfrac{115}{192} - \dfrac{1}{8} \cos{\varphi} \right) + \dfrac{a\dot{\varphi}^3}{8} \sin{\varphi} + \dfrac{g \dot{\varphi}}{8} \sin{\varphi} \overset{!}{=} 0
\end{align*}The motion does not look simple harmonic, at least for finite ##\varphi##. To investigate we can put ##\cos{\varphi} = 1## and ##\sin{\varphi} = \varphi##, and I assume also ##\dot{\varphi}^2 = 0##, which gives\begin{align*}
\ddot{\varphi} + \dfrac{12g}{91a} \varphi = 0 \implies \Omega &= \sqrt{\dfrac{12 g}{91 a}} \\
T &= \pi \sqrt{\dfrac{91a}{3g}}
\end{align*}However in the solution manual they write ##T = \pi \sqrt{29a/g}##?
]
