- #1
thevinciz
- 49
- 1
Homework Statement
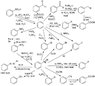
A sufficiently small steel smooth spherical object is initially at rest at point A on the smooth surface of a finite parabolic curve AOB in a vertical plane as shown in Fig.3-1, where the point O stands for the bottom point, the tangential line at O being horizontal, the vertical height at point A (relative to O) being 2H, the vertical height at point B (relative to O) being H, the horizontal distance between B and O being 2H. At time t=0, the object is released slowly to move down frictionlessly along the curve toward point B, where it departs into the air. The effects of the rotation of the object around its center is assumed to be negligible.
(1) The slope angle of the object orbit measured from the horizontal level just after release in the air at B is
(2)Finally the object will reach a point C with the same horizontal level as that at O. The necessary time to travel to C from B is
Homework Equations
The Attempt at a Solution
For the question1, I got the speed at point B = square root of 2gH. Then I named the angle which question asked, θ, so I got speed in y-axis and x-axis, (square root of 2gH)sinθ and (square root of 2gH)cosθ ,respectively. And I tried to find the θ by combined the 2 speed into triangle picture but it was not a correct way to find the answer.
(I only know that the correct answer is 45°)
For the question2, I used a projectile range's formula R=v^2sin2θ/g and I got R=2H. From S=vt (x-axis), 2H= (square root of 2gH)cos45°t, then I got time = (square root of H/g)*2 but it was wrong answer because the correct answer is (1+square root of 3)*square root of H/g.
Attachments
Last edited by a moderator: