Parametrizing Position of a Spyrograph

  • Thread starter greenspring
  • Start date
  • Tags
    Position
In summary, the conversation is about parametrizing the position of a spyrograph and finding the appropriate equations for its design. The spyrograph has 63 gears on the gear that goes around and 72 gears on the main spyrograph. The equations used involve sin and cos and the equation for finding lengths of arcs using radius and angle. After some trial and error, the equations were found to be x=cost + cos8t and y=sint + sin8t. The conversation also references a Wikipedia page for further information on spyrographs.
  • #1
greenspring
3
0

Homework Statement


I'm supposed to parametrize the position of a spyrograph.

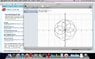
Say you have a spyrograph and you make a design like this:
Spirograph+3.jpg


In my problem, the spyrograph had to come around 8 times in order to complete its path.
There are 63 gears on the gear that went around and 72 gears on the spyrograph. I can try to find a picture if anyone doesn't understand what I'm talking about or doesn't know what a spyrograph is.



Homework Equations


sin and cos could be used, and possibly the equation for finding lengths of arcs when you know the radius and angle:
arc length=radius(theta in radians)


The Attempt at a Solution



I tried putting it in terms of theta, but I don't know why or how to do that since they're not circles. Unless maybe the whole thing is the circle, but still, why would cos and sin come into play? And how does the amount of gears come into play?

I'd very much appreciate your help please! And sorry if this is is more Calc than PreCalc.
 
Physics news on Phys.org
  • #2
Hi again! I found out using guess and check that the equation to the picture attached to this reply is x=cost + cos8t and y=sint + sin8t. Could someone please tell me how to get from the picture to that equation? Please!
 

Attachments

  • Screen shot 2010-09-27 at 4.39.53 PM.jpg
    Screen shot 2010-09-27 at 4.39.53 PM.jpg
    53.7 KB · Views: 427
  • #4
Thank you so much! It makes more sense now.
 

FAQ: Parametrizing Position of a Spyrograph

What is the purpose of parametrizing the position of a spirograph?

Parametrizing the position of a spirograph allows us to describe the exact location of the spirograph at any given time. This is important for studying the movement and patterns created by the spirograph.

How is the position of a spirograph parametrized?

The position of a spirograph is typically parametrized using polar coordinates, where the distance and angle from the center point are used to describe its location. These coordinates can then be converted into Cartesian coordinates for easier visualization.

Can the position of a spirograph be parametrized in other ways?

Yes, the position of a spirograph can also be parametrized using complex numbers or parametric equations. However, polar coordinates are the most commonly used method.

How does parametrizing the position of a spirograph help in creating different patterns?

Parametrizing the position of a spirograph allows us to adjust the parameters (such as the distance and angle) to create different patterns. By changing these parameters, we can control the size, shape, and complexity of the patterns created by the spirograph.

Is there a mathematical formula for parametrizing the position of a spirograph?

Yes, there is a formula for parametrizing the position of a spirograph using polar coordinates. It is r = a + b * cos(nt) + c * cos(mt), where 'r' is the distance from the center point, 'a' is the radius of the fixed circle, 'b' and 'c' are the radii of the moving circles, and 'n' and 'm' are the ratios of the number of teeth on the moving circles to the number of teeth on the fixed circle.

Similar threads

Finding parametric representation of a surface
Replies
10
Views
2K
Shortest Distance Between Two Latitude/Longitude Coordinates
Replies
4
Views
3K
Could someone explain this hypocycloid question?
Replies
18
Views
2K
B Question about the limits of integration in a change of variables
Replies
2
Views
1K
Finding the Center and Radius of a Circle with Complex Numbers and Loci
Replies
6
Views
3K
Deriving the Matrix for a 3 dimensional rotation
Replies
9
Views
2K
Correct Parametrization for Calculating Area of a Tube?
Replies
2
Views
933
Side lengths of inscribed triangle
Replies
30
Views
4K
A Finding Minimal Mean Distance Curves on the Unit Sphere
Replies
2
Views
2K
Deriving the sum of sin and cos formula
Replies
2
Views
2K

Hot Threads

Recent Insights

Back
Top