- #1
END
- 36
- 4
What's the best approach to solving the partial-fraction decomposition of the following expression?
$$\frac{1}{(a-y)(b-y)}$$
The expression is not of the following forms:
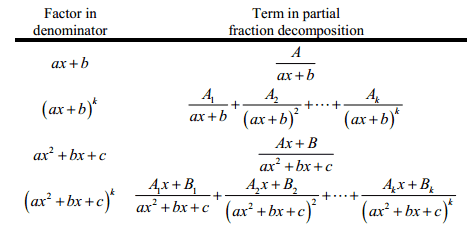
But I know the solution is
$$= \frac{1}{(a-b)(y-a)}-\frac{1}{(a-b)(y-b)}$$
$$\frac{1}{(a-y)(b-y)}$$
The expression is not of the following forms:
But I know the solution is
$$= \frac{1}{(a-b)(y-a)}-\frac{1}{(a-b)(y-b)}$$