Particle confined in 3D box - quantum states

In summary, the concept of a particle confined in a three-dimensional box, also known as a "particle in a box" model, describes how a quantum particle behaves within a defined spatial region where it is restricted by infinite potential barriers. This model leads to quantized energy levels and wave functions, with the allowed states being determined by the dimensions of the box and the boundary conditions. The solutions to the Schrödinger equation for this system result in discrete energy levels and corresponding wave functions, illustrating fundamental concepts of quantum mechanics such as quantization and superposition.
  • #1
greg997
109
2
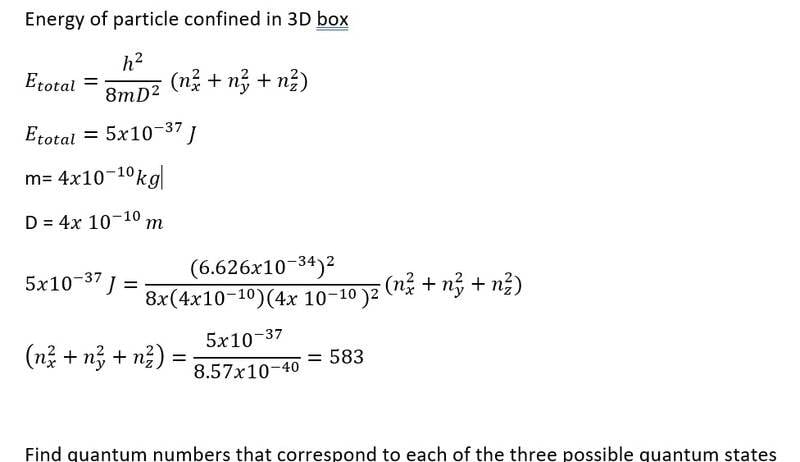
Homework Statement
Find quantum numbers for each of three possible quantum states
Relevant Equations
E= (( h^2)/(8mD) ) (nx^2_ny^2, nz^2)
Hi Everyone.
I hope someone can point me in right direction. I am struggling to work this out . If it was 1d confinement the calculated n number would be the energy level. So for example n= 3, means that quantum number is n= 3 and there is 3 possible quantum states. Is that correct?

With 3D box i am getting confused what values nx , ny, nx can have for the E given.
1.jpg
 
Physics news on Phys.org
  • #2
I asked Wolfram as shown
1714694120082.png

There seems no integers to satisfy the relation. In neighbor, (2,3,27) satisfies
[tex]a^2+b^2+c^2=582[/tex] and (2,2,24),(6,8,22) satisfies
[tex]a^2+b^2+c^2=584[/tex] where I excluded (0,10,22) which includes physically prohibited 0.
 
Last edited:
  • #3
Thank you for that. What would be the conclusion, interpretation of such solution? - No such energy levels exists?
 
  • #4
I am surprised that, with 1 digit of precision in the given variables, the search is limited to ##\sum n^2 = 583\quad ## :rolleyes:

##\ ##
 
  • Like
Likes Steve4Physics
  • #5
greg997 said:
Thank you for that. What would be the conclusion, interpretation of such solution? - No such energy levels exists?

In a narrow sense of mathematics, you are right. But in physics almost all the numbers in calculation is approximate. I am afraid that thinking of integer 583 just is not practical.
 
  • #6
greg997 said:
If it was 1d confinement the calculated n number would be the energy level. So for example n= 3, means that quantum number is n= 3 and there is 3 possible quantum states. Is that correct?
Not quite. Given a particular value of energy, if you found n=3 that would mean the system is in the n=3 state. There is only one such state for a simple '1D particle in a box' and there are an infinite number of other states (each with its own unique energy value).

greg997 said:
With 3D box i am getting confused what values nx , ny, nx can have for the E given.
View attachment 344371
The box is about the size of a (large) atom.
The mass is about ##10^{15}## times bigger than the mass of a large atom.
The value of energy is remakably small.
So I’m wondering if you have the correct data/units?

And, as others have pointed out, an energy of ##5 \times 10^{-37}## J is precise to only 1 significant figure. This would mean the energy is between ##4.5 \times 10^{-37}## J and ##5.5 \times 10^{-37}## J. Similarly for other values.
 
Last edited:

FAQ: Particle confined in 3D box - quantum states

What is a particle confined in a 3D box?

A particle confined in a 3D box refers to a theoretical model in quantum mechanics where a particle, such as an electron, is confined within a cubic or rectangular potential well. The walls of the box are considered to be impenetrable, meaning the particle cannot escape the confines of the box, leading to quantized energy levels and specific quantum states.

What are the quantum states of a particle in a 3D box?

The quantum states of a particle in a 3D box are characterized by the solutions to the Schrödinger equation under the boundary conditions imposed by the walls of the box. These states are labeled by three quantum numbers (n_x, n_y, n_z), corresponding to the dimensions of the box. The energy levels are quantized and given by the formula E = (h²/8m) * (n_x²/L_x² + n_y²/L_y² + n_z²/L_z²), where h is Planck's constant, m is the mass of the particle, and L_x, L_y, and L_z are the lengths of the box in each dimension.

How does the size of the box affect the quantum states?

The size of the box significantly affects the quantum states and energy levels of the particle. As the dimensions of the box increase, the energy levels become closer together, leading to a smaller energy difference between states. Conversely, a smaller box leads to larger energy differences and higher energy levels. This size dependency illustrates the quantization of energy and the wave nature of particles in confined systems.

What are the implications of the particle in a 3D box model?

The particle in a 3D box model serves as a fundamental example in quantum mechanics, illustrating key concepts such as quantization, wave functions, and boundary conditions. It provides insights into more complex systems, including quantum dots, nanostructures, and other confined particles in various fields of physics and engineering. The model also helps in understanding phenomena like electron behavior in solids and the principles of quantum confinement.

Can the particle in a 3D box model be applied to real-world systems?

Yes, the particle in a 3D box model can be applied to various real-world systems, particularly in the fields of nanotechnology and solid-state physics. For instance, it can describe the behavior of electrons in quantum dots, which are semiconductor particles that confine electrons in three dimensions. This model aids in the design and understanding of devices such as transistors, lasers, and other electronic components where quantum effects are significant.

Similar threads

Degeneracy of the energy level
Replies
6
Views
2K
What are the possible quantum states of an electron relaxing from n=4 to n=3?
Replies
1
Views
928
(QM) Number of states with Energy less than E
Replies
6
Views
2K
Help Calculating probability between 2 limits for the ground state
Replies
28
Views
1K
Vibrational Levels in Molecular Electronic State
Replies
1
Views
1K
How Do Energy Levels Work for Electrons in a 1D Box?
Replies
30
Views
6K
Electron confined in a one dimensional box
Replies
1
Views
3K
Relationship between k and E when deriving the density of states
Replies
1
Views
1K
How do you prove ##\lambda = \frac {2L} {n} ## given only L?
Replies
3
Views
484
Python Thermal lattice Boltzmann model ignoring source term -- python code help please
Replies
1
Views
2K

Hot Threads

Recent Insights

Back
Top