Particle in a box question in classical mechaincs

[Taylor_1989]

Hi guys, I am having an issue with what my lecture is saying in these slides, I have attched my slides below.

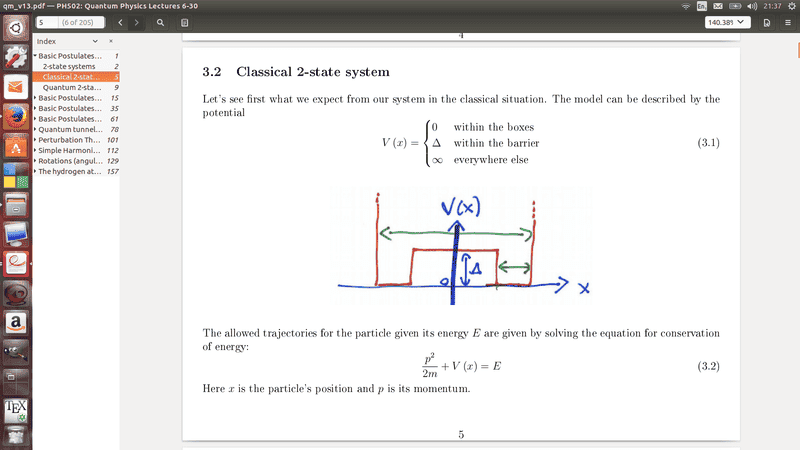
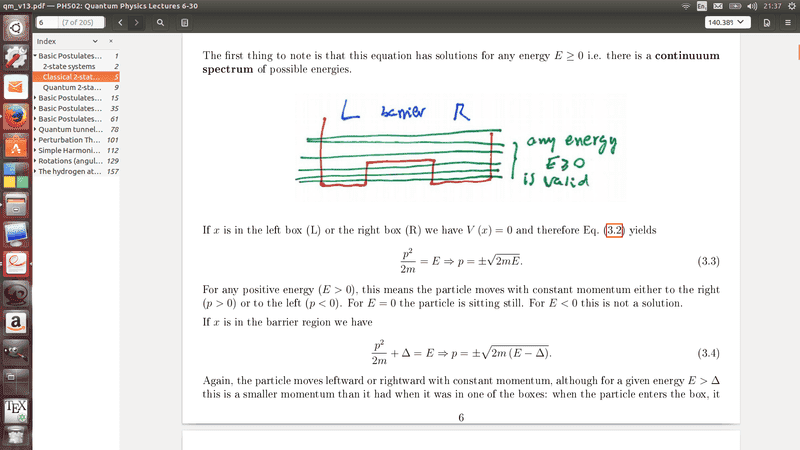
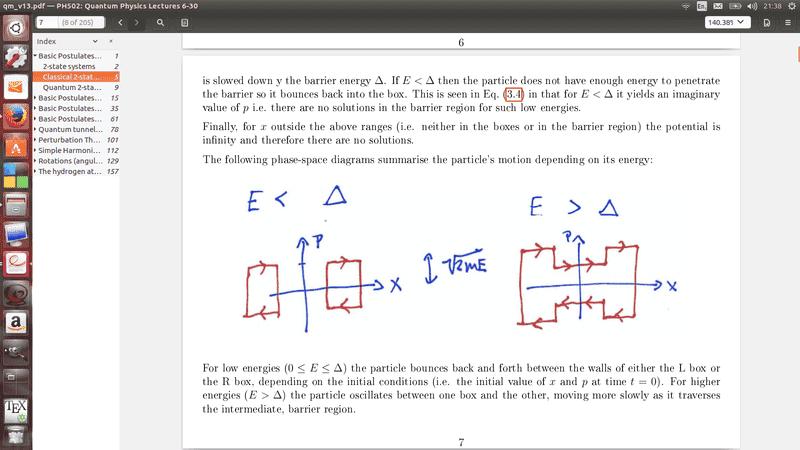
Here is my issue. I am very confused by the $E<\Delta$ Beacuse I can't see how this has any momentum as it would produce an imagery number. And ye say that $E<\Delta$ cannot be a solution yet on these diagrams he show the particle has momentum, at E=0 which can't be correct. I know I have this back to front, because he also mentions that at $E>\Delta$ that is has less momentum than but I just can't see how, could someone please explain to me what my lecture is conveying here.

 

[ehild]

Here is my issue. I am very confused by the $E<\Delta$ Beacuse I can't see how this has any momentum as it would produce an imagery number. And ye say that $E<\Delta$ cannot be a solution yet on these diagrams he show the particle has momentum, at E=0 which can't be correct. I know I have this back to front, because he also mentions that at $E>\Delta$ that is has less momentum than but I just can't see how, could someone please explain to me what my lecture is conveying here.

If E<Δ the particle is confined in one of the boxes, where the potential is zero.

 

[Taylor_1989]

This is confusing, if I look at the equation it tell me that momentum dose not exist it imaginary. I can't seem to think how this particle is moving inside the box.

I mean if I look over this again he states that at 0 poteinal the particle is in the right of left box, so the particle as I see it is moving to the left and right at the bottom of one of the boxes. But how if E=0 the $P=\sqrt(2m(E-\Delta))$ then the solution give an imaginary value so tho I can see it physically I can see how it is relating to the equation.

Is there a link to either a website or video that will help me understand this concept?

Also where is this energy barrier I can't see how this particle is moving from box to to box

 

[Taylor_1989]

Okay so I have been thinking about this a bit more. I have attched photos of my workings, and tryed to explain best to what I think is going on, I know normally it not great to post photo but I couldn’t draw a decent enough diagram to convey what I was thinking.

 

[ehild]

This is confusing, if I look at the equation it tell me that momentum dose not exist it imaginary. I can't seem to think how this particle is moving inside the box.

I mean if I look over this again he states that at 0 poteinal the particle is in the right of left box, so the particle as I see it is moving to the left and right at the bottom of one of the boxes. But how if E=0 the $P=\sqrt(2m(E-\Delta))$ then the solution give an imaginary value so tho I can see it physically I can see how it is relating to the equation.

Remember, the equation is p²/2m +V(x) =E
There are walls of infinite high at x1 and x4. There is a barrier between x2 and x3. The function is defined between x1 and x2, outside it is infinite.

x1<x ≤ x2 V(x) = 0
x2 <x ≤ x3 V(x) = Δ
x3 < x ≤ x4 V(x) = 0

If E < Δ the particle can not exist in the interval x2 <x ≤ x3. It is confined "inside" one of the boxes, either between x1 and x2 or between x3 and x4.

Also where is this energy barrier I can't see how this particle is moving from box to to box

If E ≥ Δ the particle can exist in the whole interval x1 <x ≤ x4, only its kinetic energy changes above the barrier. It is not "inside" any boxes

 

[ehild]

Okay so I have been thinking about this a bit more. I have attched photos of my workings, and tryed to explain best to what I think is going on, I know normally it not great to post photo but I couldn’t draw a decent enough diagram to convey what I was thinking.

View attachment 216869

View attachment 216870

View attachment 216871

The drawing is right, but I can not read your handwriting.

 

[Taylor_1989]

The drawing is right, but I can not read your handwriting.

Sorry, from your pervious comment, and a couple more hours, I realized what was going on. Thank you for the advice.