Particle Kinematics with Friction

[aaronfue]

Homework Statement

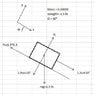
A 1.5-lb brick is released from rest A and slides down the inclined roof. If the coefficient of friction between the roof and the brick is μ=0.3, determine the speed at which the brick strikes the gutter G.

The Attempt at a Solution

These are the equations that I got after setting up my FBD attached:

Fr(friction force) = 0.3*Normal force
ƩFx = 0.04658a_x = -Fr + 1.5sin30°
a_x = -11.81 $\frac{ft}{s^2}$

Then using the kinematic equation v² = v_o² + 2a(s-s_o)

v_o = 0
v² = 2(11.81)(15)
v = 18.82$\frac{ft}{s}$

But that is not the correct velocity. I know I'm missing something. I just can't find it! The correct answer was 15.23 ft/s.

 

[tms]

Your general approach seems sound, but it's hard to tell where you went wrong because you left so many steps out, and because you plugged in the numbers so early (when you do that you lose information about where the numbers came from).

I would suggest that you do it again using symbols instead of numbers. Pay particular attention to the frictional force.

 

[voko]

First of all, you do not really care what the mass or weight of the brick is.

$$N = mg \cos \alpha \\ Fr = - \mu N = - \mu mg \cos \alpha \\ ma = mg \sin \alpha + Fr = mg \sin \alpha - \mu mg \cos \alpha$$

$m$ can now be eliminated from the equation.

 

[aaronfue]

First of all, you do not really care what the mass or weight of the brick is.

$$N = mg \cos \alpha \\ Fr = - \mu N = - \mu mg \cos \alpha \\ ma = mg \sin \alpha + Fr = mg \sin \alpha - \mu mg \cos \alpha$$

$m$ can now be eliminated from the equation.

Okay. I have arrived at your final equation of:

a=gsinθ - μgcosθ (Eliminating the mass)

Now that I have the acceleration, I can plug this into my kinematic equation to obtain the final velocity as the brick completes the 15 ft or (s-s_o).

v² = v_o² + 2*(gsinθ - μgcosθ)*(s-s_o)
v_o² = 0, since the brick started at rest.

If I were to plug in my values now, this would not be my answer.

 

[CAF123]

If I were to plug in my values now, this would not be my answer.

I get the required answer using that equation.
Alternatively, you can do this by energy methods to get:(Take zero of potential at start of gutter) $$mgh - F_fd = \frac{1}{2}mv^2\,\Rightarrow\,v^2 = 2(gh - \mu g\cos \theta d),$$ to get the same answer.

 

[aaronfue]

I get the required answer using that equation.
Alternatively, you can do this by energy methods to get:(Take zero of potential at start of gutter) $$mgh - F_fd = \frac{1}{2}mv^2\,\Rightarrow\,v^2 = 2(gh - \mu g\cos \theta d),$$ to get the same answer.

You got 15.23 ft/s? I've been using two calculators (TI-89 & TI-83 plus) and have still not gotten that answer. I have made sure to be in the correct mode as well (degrees) but I'm not sure what my problem is. Would you be able to explain your calculations on your calculator or tell me what I'm doing wrong?

 

[CAF123]

You got 15.23 ft/s? I've been using two calculators (TI-89 & TI-83 plus) and have still not gotten that answer. I have made sure to be in the correct mode as well (degrees) but I'm not sure what my problem is. Would you be able to explain your calculations on your calc?

So you are in the correct mode. What value are you taking for $g?$. I converted everything into the more conventional units we use today. Would that be your problem?

 

[aaronfue]

So you are in the correct mode. What value are you taking for $g?$. I converted everything into the more conventional units we use today. Would that be your problem?

I'm using 32.2ft/s² since we are using ft in the problem. I'll try to convert everything to m/s and 9.81m/s².

 

[CAF123]

I'm using 32.2ft/s² since we are using ft in the problem. I'll try to convert everything to m/s and 9.81m/s².

That would also be valid. What number are you getting?

 

[aaronfue]

That would also be valid. What number are you getting?

For a second I was getting 11.20210. Now I am getting 21.799. I've attached a screenshot of my calculation.

Wait...I've got it!

I guess my order of operations was not going well. And I forgot to multiply μ*g.

I only had μcosθ.

The attachment shown was after calculating the sin and cos separately, then multiplying by 15, and then by 2. The square root of 232.025838 gives me my answer.