- #1
pompey
- 6
- 0
Homework Statement
(This is from Giancoli 5th ed book, pg 500 problem 49)
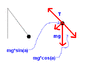
A point charge m=1.0g at the end of an insulating string 50cm long is in equilibrium in a uniform electrical field E=9200 N/C, when the pendulum is 1.0cm high. If the field point to the right, what is the magnitude of the point charge?
Homework Equations
F=Eq
The Attempt at a Solution
I think the solution is q = tan(theta)*m*g/E
where theta = arccos(.49/.50) and m=1.0g and g = 9.8m/s^2
However, I found it to be q = cos(theta)*sin(theta)*m*g/E, which I know to be wrong.
I've had trouble with free force diagrams for pendulums, and I can draw one out but I can't find the force component in the X direction. If T = tension in the string, then the component in the X direction should be sin(theta)*T. T should equal m*g*cos(theta), so shouldn't the total force in the X direction be sin(theta)*cos(theta)*m*g