- #1
sbaker8688
- 29
- 6
- TL;DR Summary
- Why doesn't the Penrose diagram of a black hole look like this?
I'm no expert in this stuff, and perhaps I don't understand these diagrams, but having said that, I don't understand why the typical Penrose diagrams I see of black holes look the way that they do. They all have a 45 degree (light speed) angle for the event horizon of the black hole, and they always seem (to my understanding) to depict the black hole as growing over time, and/or taking up huge amounts of space (like, infinite).
Here is my (mis?) conception of what a black hole Penrose diagram should look like. Assume this thing just formed at time and space coordinates 0,0 (the present). The event horizon has a width W. I've colored the black hole red in the diagram. To me, it should basically be a shaded box going straight up to infinity.
Explain why this is wrong. Thanks.
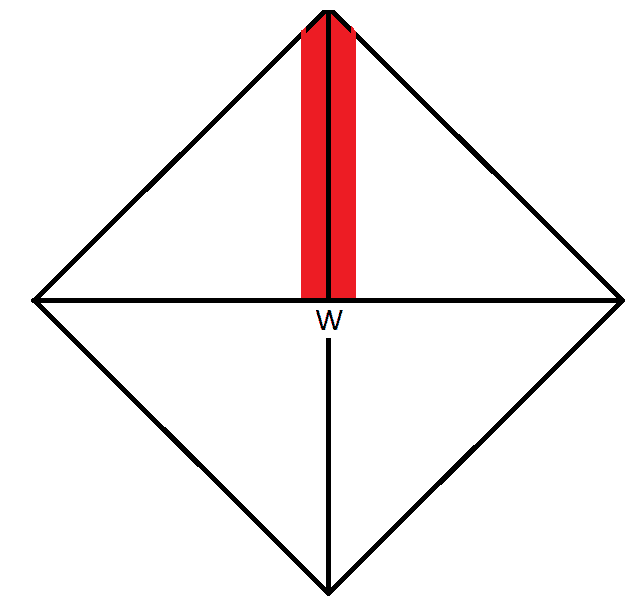
Here is my (mis?) conception of what a black hole Penrose diagram should look like. Assume this thing just formed at time and space coordinates 0,0 (the present). The event horizon has a width W. I've colored the black hole red in the diagram. To me, it should basically be a shaded box going straight up to infinity.
Explain why this is wrong. Thanks.
