Penrose Diagram for the Kerr Black Hole

[Clauslism]

Hey Guys,
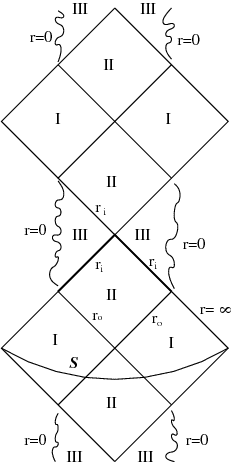
so i was reading Hawking&Ellis a bit and still encounter always problems with the Penrose-Diagrams. Looking at the Penrose-Diagram for the rotating Kerr-Black hole (just one illustrating picture at the end) i come up the following question:

Why are there TWO regions III and III ?

In each of these regions we encounter a ring singularity. Yet shouldn"t it be the same singularity? Are regions somehow to be identified with each other? I don't think so, since the Interpretation of region I and I eg. is, that both are to be understand as different parts of the same universe - or some understand them even as two separate universes.
So are there indeed two different singularities? If so: how would force them to have the same physical properties? Conctrely: Could there be a stationary solution where one singularity has different charges and angular momentum, then the other?

And how would you describe the trajectories of observers who want to go to either one of the to singularities? "How should i travel to reach the singularity i want?"

Thanky you very much for your help! :)

http://inspirehep.net/record/841642/files/rnpd2.png

 

[PeterDonis]

Why are there TWO regions III and III ?

For the same reason there are two regions I and I, and two regions II and II (and in fact an infinite number of "blocks" of this form): because when you work out the math, that's what comes out. Remember that this is an idealized mathematical solution to the Einstein Field Equation; it is not believed to describe an actual rotating black hole that formed from some other rotating object. In the idealized mathematical solution, as long as $r$ is greater than zero and less than infinity, you can extend the solution further; and $r$ is nonzero and finite at the boundaries between the I and II regions and between the II and III regions.

are there indeed two different singularities?

Yes. Each of the regions in the diagram is a distinct region, and each of the singularities is a distinct singularity.

how would force them to have the same physical properties?

The overall symmetry of the spacetime. The Kerr solution has only two free parameters, the hole's mass and angular momentum, and they are the same everywhere in a given solution (i.e., everywhere in the diagram). Those parameters determine the properties of the singularities, just as they determine the properties of the spacetime as a whole.

 

[Clauslism]

Thank you for your answer!

as long as rr is greater than zero and less than infinity, you can extend the solution further;

Yeah, i know the idea behind the maximal analytic extension... but r being great than zero is no requirement, isn't it? For the ring singularity you extend also to negative values of r, which is where the regions come into play, which finally would allow closed timelike curves. Right?

Remember that this is an idealized mathematical solution to the Einstein Field Equation; it is not believed to describe an actual rotating black hole that formed from some other rotating object.

Of course: even putting an obsver in this framework destroys it. But regard it as a sort of an "thought experiment": You have this eternal rotating BH and now an observer carring angular momentum would fall into the singularity! If these are two different regions and he would end his life in only of the singularties, he could only change one of the singularities. In an idea like the Penrose Process wouldn't i be able to extract TWICE the energy from the BH, because there are two singularites carrying the same angular momentum?

And i also have this problem of imaging, "where" these singularties are located/and these regions III are to be differentiated from each other. with this i mean: are you somehow able to choose which region III you encounter?
My understanding is the following: the curves of constant radii in region II are the "tangential horizontal" lines (going from the outer event horizon r_o to the inner chauchy surface r_i). Then the "tangential vertical lines" describe some sort of curves which are constant in "time"? But to call it "time" seems to be somewhat wrong, since in region II you could now be able in principle to choose (?) wheter you send signals to increasing or decreasing values of t! So how would you call it?
And then again the question: If i try to imagine me falling behind r_o what "should i do" to travel to the next universe (=reaching the new white-hole region II)? (Because eg reaching this universe of negative values r, is achieved by traveling "through the ring" - and essentially i am looking for an "easy picture" like that for crossing r_i to the next universe)

 

[PeterDonis]

r being great than zero is no requirement, isn't it?

Yes, you're right, for Kerr spacetime $r$ can go all the way to minus infinity in the regions "inside" the ring singularities. These regions are not marked on the diagram; if they were, they would be little triangles extending from the $r = 0$ singularities. In the regions marked I, II, and III on the diagram, $r$ is greater than zero.

You have this eternal rotating BH and now an observer carring angular momentum would fall into the singularity! If these are two different regions and he would end his life in only of the singularties, he could only change one of the singularities. In an idea like the Penrose Process wouldn't i be able to extract TWICE the energy from the BH, because there are two singularites carrying the same angular momentum?

No to both of these. The angular momentum is not a property of the singularities; it's a property of the spacetime as a whole. In a process where an object falls in and changes the angular momentum, as far as an observer at infinity is concerned, the angular momentum was "changed" from the start; the total angular momentum of the spacetime, as viewed from infinity, always included the angular momentum of the object as well as that of the hole. As far as observers at finite altitudes are concerned, if you have to pick a point at which the angular momentum of the hole "changes", it is when the infalling object crosses the outer horizon; so if you insist on localizing the hole's angular momentum, the best place to think of it being localized is at the outer horizon.

 

[PeterDonis]

are you somehow able to choose which region III you encounter?

Yes. In practice I'm not sure how you would control your trajectory to do that (i.e., what measurements you would make to ensure that you ended up in, say, the left region III as opposed to the right one), but in principle you could do so.

the curves of constant radii in region II are the "tangential horizontal" lines (going from the outer event horizon r_o to the inner chauchy surface r_i)

If by "constant radii" you mean "constant radial coordinate $r$", those curves are not straight lines on this diagram, except for a few special cases. In region I, curves of constant $r$ start at the bottom corner and end at the top corner; smaller values of $r$ curve inward towards the center of the diagram (the corner where two region I's and two region II's meet), and larger values of $r$ curve outward towards the edge of the diagram. In region II, curves of constant $r$ start at the right corner and end at the left corner; larger values of $r$ curve downward towards the center of the diagram, and smaller values of $r$ curve upward towards the top corner of region II (where two region II's and two region III's meet). In region III, curves of constant $r$ look like they do in region I. (They also do in the region of negative $r$ on the other side of the singularities.)

If i try to imagine me falling behind r_o what "should i do" to travel to the next universe (=reaching the new white-hole region II)?

Just keep falling. Inside region III, as you get closer to the ring singularities, gravity becomes "repulsive"--a freely falling object will stop short of the singularity and start moving outward (increasing $r$) again, eventually "falling" into the region II above region III, and then into one of the region I's above that (you can choose which one).

 

[Clauslism]

No to both of these. The angular momentum is not a property of the singularities; it's a property of the spacetime as a whole

That's true, its describing the spacetime as a whole and strictley mathematical spoken we are done. But if you for example take the Penrose Process, you assume that this approximatley describes only a part of our universe and we can come from far away with particles carrying angular momentum (if you would not, then how should this idea of taking energy from the BH work? You send in particles which reduce the angular momentum of the BH, which is only possible if you make a distinction between the BH angular momentum and the angular momentum of the particle). So from physics point of view, we assume we have a BH carrying angular momentum and a particle carrying a different angular momentum. As soon as the particle enters the event horizon, we don't know wheter it travels to the next universe or ends its live in one of the singularties. If it travels to the next universe however, the angular momentum of the BH itself should not change! So the only possibiliy to make the Penrose Process realistic seems to me, by postulating that the particle ends its life in one of the singularties...
I know that these are assumptions which violate the mathematical structure behind the Kerr solution. Yet is this not the same thing one does to describe the Penrose process? (saying you have little particles whose influence on the metric can be neglected in comparrison to the influence of the BH?

 

[Clauslism]

Just keep falling. Inside region III, as you get closer to the ring singularities, gravity becomes "repulsive"

That's interessting: i read about this before, but assumed it must be wrong. I was always thinking, that the regions where gravity becomes repulsive are the regions of negative r (the regions inside the ring singularity). Can you please give me a rough scetch how to see from the metric (or however you do it) where gravity starts to get repulsive? I would very much appretiate it, because i was never able to find it anywhere!

 

[PeterDonis]

if you for example take the Penrose Process, you assume that this approximatley describes only a part of our universe

If we are talking about the Penrose process, or any process in which the angular momentum of the black hole changes, we are no longer talking about the complete Kerr spacetime. That's why I was careful to point out that the Penrose diagram you gave is for an idealized solution; it's idealized not just in the sense that it is perfectly symmetrical, but in the sense that it can't participate in any real process, because none of its properties can ever change. It can't even have formed by any real process, because it's vacuum everywhere. Any real black hole, formed by a real process, would not have the singularities or the other universes--in fact it probably wouldn't even have the inner horizon, because quantum gravity effects would probably become large enough to make the classical GR solution invalid before the inner horizon was reached. So it's simply not consistent to use the Penrose diagram you gave to analyze the Penrose process, or any other process that changes the hole's properties.

As soon as the particle enters the event horizon, we don't know wheter it travels to the next universe or ends its live in one of the singularties.

Again, if the particle falling in can change the angular momentum (or mass) of the black hole, then the idealized Penrose diagram you gave no longer applies, and the real hole would not have the singularities or the other universes, for the reasons given above. The only kind of particle that you can imagine as traveling into region III and having a choice of hitting one of the singularities or traveling to one of the other universes is a "test particle", with zero mass and zero angular momentum, so that it doesn't change the hole's properties at all.

 

[Clauslism]

-in fact it probably wouldn't even have the inner horizon, because quantum gravity effects would probably become large enough to make the classical GR solution invalid before the inner horizon was reached

I don't want to include QG at all in this picture - so many different theories and none of them tells us up to today anything about the interior of the BH.
But of course the formal statement would be to say, that everything inside the event horizon can never be observed by us and thus cannot be described properly.
Let us just make the obviously wrong assumption GR would describe the spacetime completely.

So it's simply not consistent to use the Penrose diagram you gave to analyze the Penrose process, or any other process that changes the hole's properties

But what do Physcists use to describe something like the Penrose Process? Following your argumentation (and i understand them and agree with them in general) they cannot use the Kerr-solution! But as far as i can find it in the literatur the always work with the Kerr-spacetime - so where is the mistake? How would one implement the Penrose Process in a mathematical correct formulation?And could you please just briefly comment on the topic of repulsive gravity? How does one sees where the gravity becomes repulsive? :)

Thank you very much and have a happy new year!

 

[PeterDonis]

what do Physcists use to describe something like the Penrose Process Following your argumentation (and i understand them and agree with them in general) they cannot use the Kerr-solution!

Yes, they can; they just can't use the inner horizon and the region inside it. But you don't need to use that region to analyze the Penrose process; you just need the ergosphere and the outer horizon. You only need the inner regions if you want to analyze what happens to an object after it falls through the outer horizon; but you don't need to know that to analyze the Penrose process, at least the part that is observable to us outside the outer horizon.

It's true that the Penrose process requires a black hole that changes mass and angular momentum, and strictly speaking, Kerr spacetime can't model that. However, Kerr spacetime still provides a very good approximation for a process that changes the hole's mass and angular momentum by a very small amount--for example, an object massing a few tons falling into a black hole a few times the mass of the Sun. This process can be modeled to a very good approximation by using Kerr spacetime with the original mass and angular momentum of the hole, and then, after the object falls in, just replacing it with Kerr spacetime with the new mass and angular momentum of the hole. The change is so small, and happens so fast on the time scale of the hole, that there is no need to model the detailed dynamics of the change. I should have clarified that in my earlier post.

 

[PeterDonis]

How does one sees where the gravity becomes repulsive?

I don't know of any easy way to read this off from the metric. In fact, exactly where gravity becomes repulsive is rather complicated, because Kerr spacetime is not spherically symmetric, it's only axially symmetric. The Penrose diagram you gave only applies in the equatorial plane of Kerr spacetime, and IIRC, in that plane gravity actually is only repulsive for $r < 0$. But outside the equatorial plane, IIRC there are positive values of $r$ for which gravity is repulsive. I'll have to check the details when I get a chance; the computations are rather tedious.

 

[stevebd1]

And could you please just briefly comment on the topic of repulsive gravity? How does one sees where the gravity becomes repulsive?

While there is a debate about the validity of the inner (Cauchy) horizon, by looking at Killing surface gravity equation for Kerr black holes, you can see that gravity would be negative at the inner horizon for an object dropped from rest at infinity with zero angular momentum (ZAMO)-

$$\tag{1}\kappa_\pm=\frac{r_\pm-r_\mp}{2(r_\pm^2+a^2)}$$

where $r_\pm=M\pm\sqrt(M^2-a^2)$ or in the case of Kerr-Newman, $r_\pm=M\pm\sqrt(M^2-a^2-Q^2)$ where $a^2+Q^2\leq M^2$

for an equation of the gravity locally (i.e. taking into account relativity) for a ZAMO, the following equation applies (in the equatorial plane only) for Kerr black holes-

$$\tag{2}a(n)_{\hat{r}}=\frac{M\left[(r^2+a^2)^2-4a^2Mr\right]}{\sqrt{\Delta}\,r^2(r^3+a^2r+2Ma^2)}$$

This equation is undefined between $r_+$ and $r_-$ due to the square root of $\Delta$ which becomes negative between $r_+$ and $r_-$, to get some idea of where gravity becomes negative mathmatically, you could set the equation so that you get the square root of the absolute value of $\Delta$

Note if you use $r_+$ and $r_-$ in equation (2) and multiply by the redshift $\alpha=(\sqrt{(\Delta)}\rho/\Sigma)$, you get results equal to equation (1)

For a more extended equation of the gravity locally for a Kerr black hole (i.e. beyond the equatorial plane) for a ZAMO the following applies-

$$\tag{3}\vec{a}(n)=\frac{M}{\Sigma^2 \rho^3}\left( \left[(r^2-a^2\cos^2\theta)(r^2+a^2)^2-4Mr^3a^2\sin^2\theta\right] \Delta^{-1/2}-2ra^2\sin\theta\cos\theta(r^2+a^2)\right)$$

It appears that gravity becomes negative somewhere near the Cauchy horizon, remains negative within the Cauchy horizon and then becomes positive again near the singularity.

source for equations (2) & (3)- http://arxiv.org/abs/gr-qc/0407004 page 10

 

[Clauslism]

You only need the inner regions if you want to analyze what happens to an object after it falls through the outer horizon; but you don't need to know that to analyze the Penrose process, at least the part that is observable to us outside the outer horizon.

But what is obsverable to us in region I if send a particle into the Kerr-BH? - The angular momentum of the BH. And this changes if and only if the particle ends its life in one of the singularties. If would transverse the singularties to one of the new universes, it should not change the momentum at all. So we could in principle tell what happened to the particle by measuring the angular momentum of the BH? Wouldn't this in a strange way violate the causal structure about: no information can escape?

 

[Clauslism]

Thanks stevebd1!

This equation is undefined between r+r_+ and r−r_- due to the square root of Δ\Delta which becomes negative between r+r_+ and r−r_-, to get some idea of where gravity becomes negative mathmatically, you could set the equation so that you get the square root of the absolute value of Δ\Delta

Does this mean that the accleration reaches infinity even before the singularity? Because Δ= r² + a² + Q² - 2Mr diverges at R_±. But then we would be expirence an infinite gravitational field even at the event horizon - i am missing something here, right? (Or is this again just a coordinate singularity?)

 

[stevebd1]

Does this mean that the accleration reaches infinity even before the singularity? Because Δ= r² + a² + Q² - 2Mr diverges at R_±. But then we would be expirence an infinite gravitational field even at the event horizon - i am missing something here, right? (Or is this again just a coordinate singularity?)

Gravity does become infinite at the event horizon but this is a coordinate singularity, the radial world lines become space like between $r_+$ and $r_-$ as apposed to time like where $r>r_+$ and $r<r_-$. In the case of the Schwarzschild BH, gravity becomes infinite at the EH but the Newtonian equation for tidal forces (or gravity gradient) remains unchanged $(dg=dr2M/r^3)$ all the way to r=0 which means gravity is still working in some sense. In the case of the Kerr BH, it may be that you free fall past the outer EH and don't experience the repulsive gravity until you hit the Cauchy horizon. It's also predicted that the Cauchy horizon may be a weak singularity which increases like a Dirac delta function (i.e. no gravity gradient) which means if an object is robust enough it may pass through.

 

[PeterDonis]

The angular momentum of the BH. And this changes if and only if the particle ends its life in one of the singularties.

No, it does not. See my post #4 in this thread.

 

[PeterDonis]

a more extended equation of the gravity locally

This is supposed to be a pair of components, correct? That is, there should be a comma after the $\Delta^{-1/2}$ , indicating the start of the $\theta$ component, correct?

 

[stevebd1]

This is supposed to be a pair of components, correct? That is, there should be a comma after the $\Delta^{-1/2}$ , indicating the start of the $\theta$ component, correct?

Yes, this is correct.

 

[Clauslism]

No, it does not. See my post #4 in this thread.

When a particle with reverse angular momentum enters the event horizon, it shrinks the total angular momentum of the BH. But the "moment" it leaves the white hole region it should take its angular momentum again with it, right? So the total angular momentum would again increase (i guess this should be right, since this nothing more but time revearsal of the before mentioned process)
So following the argumentation you used in post #10 :

after the object falls in, just replacing it with Kerr spacetime with the new mass and angular momentum of the hole. The change is so small, and happens so fast on the time scale of the hole, that there is no need to model the detailed dynamics of the change

1) particle enters event horizon - we replace the Kerr spacetime with the one with the new angular momentum
2) An observer in region I can measure the new angular momentum and tell, that the object has fallen into the BH
2) particle leaves white hole region - we replace the Kerr spacetime again with the one with the old angular momentum
3) An observer in region I can measure the old angular momentum and tell, that the object has crossed to the new universe

 

[PeterDonis]

When a particle with reverse angular momentum enters the event horizon, it shrinks the total angular momentum of the BH. But the "moment" it leaves the white hole region it should take its angular momentum again with it, right?

No, because, as I said before, if you are allowing the mass or angular momentum of the hole to change, the Penrose diagram you drew does not apply. You simply cannot use that diagram, even as an idealization, to think about what happens if the mass or angular momentum of the hole changes. See further comments below.

1) particle enters event horizon - we replace the Kerr spacetime with the one with the new angular momentum

Actually, we don't "replace" the entire spacetime. What we have is a spacetime which (in the idealized case where the change happens basically "instantaneously") looks like a perturbation (because there's an object present other than the black hole) of a Kerr spacetime with one mass and angular momentum to the past of a particular spacelike hypersurface, and looks like Kerr spacetime with another mass and angular momentum to the future of that spacelike hypersurface. But in both regions (past and future of the hypersurface), it will only look like a portion of Kerr spacetime--the exterior (outside the outer horizon), and some portion of the interior, but probably not even up to the inner horizon. (There will also be an "interior" portion that represents the object that originally collapsed to form the hole.) There will be no region III, no white hole, and no other universe. (In fact there will not even be a second region I or region II.) Sorry if I wasn't clear about this in my previous post.

If you ask, why can't we have an idealized model of a black hole with region III and the white hole and the other universes that changes mass and angular momentum, ignoring the fact that physically the inner horizon and region III and the white hole and the other universes won't be there, the answer is that there is no known way to construct one, because the construction that gives you region III and the white hole and the other universes only works in the first place if the mass and angular momentum of the hole are constants, rather than functions of position in spacetime. But in the case described above, the mass and angular momentum of the hole are functions of position in spacetime, not constants; even if we can treat them as constants in each region (past and future of the hypersurface), they are different constants in each region, which makes them functions of position in spacetime. That means you can't carry through the construction that gives you the Penrose diagram you are using.

So the only step in the process (of an object falling into the hole) that can be modeled at all, even as an idealization, is step 1; the other steps simply can't be modeled, even as an idealization.