Perfectly elastic collision between two electrons in ⊥ B-field

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

The solution is,

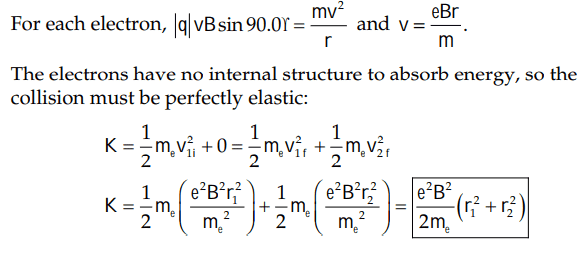
However, is the reason why they don't include electrical potential energy because the time interval for which we are applying conservation of energy over is very small so the change in electric potential energy is negligible?

Also, when they said, "electrons have no internal structure to absorb energy", would it not be more concise to say "The internal structure of electrons cannot absorb kinetic energy"?

My statement reflects that electrons do have internal structure consist of quarks which can absorb and emit energy by via photonic emission.

Many thanks!

 

[malawi_glenn]

My statement reflects that electrons do have internal structure consist of quarks which can absorb and emit energy by via photonic emission.

Protons have quarks. Electrons are, as far as we know, elementary. Perhaps this thread is a good read for you https://www.physicsforums.com/threads/how-hard-have-they-banged-on-quarks-electrons-etc.1048707/

 

[member 731016]

Protons have quarks. Electrons are, as far as we know, elementary. Perhaps this thread is a good read for you https://www.physicsforums.com/threads/how-hard-have-they-banged-on-quarks-electrons-etc.1048707/

Oh whoops, thanks for pointing that out @malawi_glenn ! Sorry for my mistake!!

 

[malawi_glenn]

And even it it where protons in this problem, you can assume that there is no change in their internal energy because we need quite high energy to change the quark configuration in them. If electrons have subparticles (preons or whatever) we still assume that the energies involved in this problem is not large enough to resolve that. It is written in the problem that they undergo elastic collision. Thus, there is no change in internal energy.

 

[member 731016]

And even it it where protons in this problem, you can assume that there is no change in their internal energy because we need quite high energy to change the quark configuration in them. If electrons have subparticles (preons or whatever) we still assume that the energies involved in this problem is not large enough to resolve that. It is written in the problem that they undergo elastic collision. Thus, there is no change in internal energy.

Thank you for your help @malawi_glenn !

 

[Steve4Physics]

However, is the reason why they don't include electrical potential energy because the time interval for which we are applying conservation of energy over is very small so the change in electric potential energy is negligible?

The final radii are measured when the electrons are far apart - so their potential energy is zero. You are determining the kinetic energy of the incident electron when the initial separation was large, so the intial potential energy was also zero. (The question could have been a bit clearer about this!)

FWIW, although electrons have no internal structure, that does not mean electron-electron collisions are necessarily elastic. Loss of (kinetic) energy can occur due to the production of EM radiation. For example that’s how an X-ray tube produces X-rays – ‘bremsstrahlung’. But you can assume the effect is negligible in this question, because you are told the collision is elastic.

 

[member 731016]

The final radii are measured when the electrons are far apart - so their potential energy is zero. You are determining the kinetic energy of the incident electron when the initial separation was large, so the intial potential energy was also zero. (The question could have been a bit clearer about this!)

FWIW, although electrons have no internal structure, that does not mean electron-electron collisions are necessarily elastic. Loss of (kinetic) energy can occur due to the production of EM radiation. For example that’s how an X-ray tube produces X-rays – ‘bremsstrahlung’. But you can assume the effect is negligible in this question, because you are told the collision is elastic.

Ok thank you for your help @Steve4Physics !