Perturbation theory and the secualr equation for double degeneration

[mcas]

Homework Statement

Determine the correction to the eigenvalue in the first approximation and the correct functions in the zeroth approximation, for a doubly degenerate level.

Relevant Equations

$det|V_{nn'}-E^{(1)}\delta_{nn'}|=0$
$\sum_{n'} (V_{nn'}-E^{(1)}\delta_{nn'})c_{n'}^{(0)}$

I've been assigned to do a problem from Landau which you can read below:

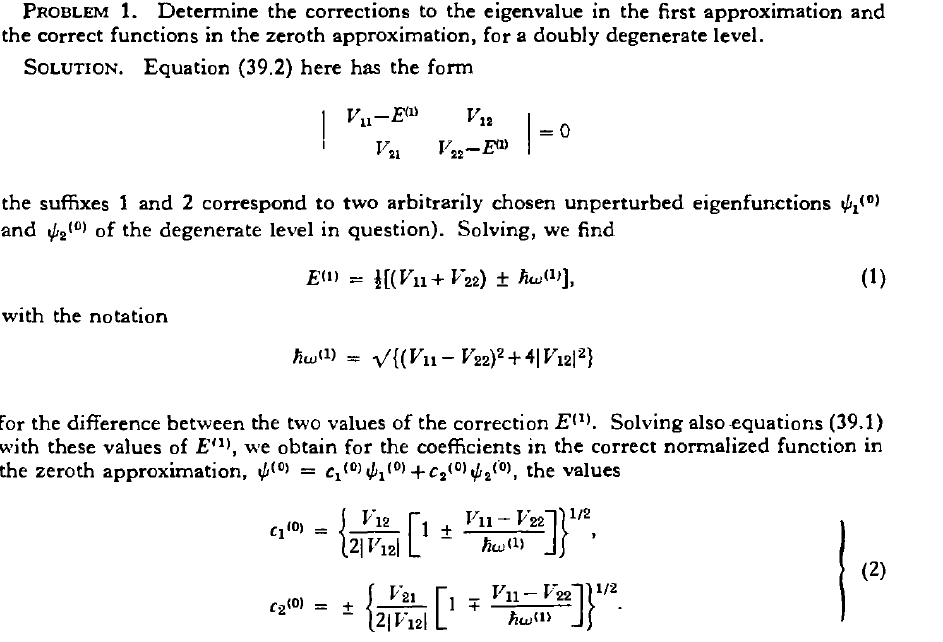
I have no problem with finding the energy. Then I write down the equations:
\begin{equation*}
\begin{cases}
(V_{11}-E^{(1)})|c_1|e^{i\alpha_1} + V_{21}e^{i\alpha_2}|c_2| = 0\\
V_{12}e^{i\alpha_1}|c_1| + (V_{22}-E^{(1)})|c_2|e^{i\alpha_2} = 0
\end{cases}
\end{equation*}

\begin{equation*}
\begin{cases}
(V_{11}-E^{(1)})|c_1|e^{i\alpha_1} = V_{21}e^{i\alpha_2}|c_2|\\
V_{12}e^{i\alpha_1}|c_1| = (V_{22}-E^{(1)})|c_2|e^{i\alpha_2}
\end{cases}
\end{equation*}
There is no negative sign because I will include it in phases as $e^{i\pi}$.
Because $V_{21}=V_{12}^*$, I can write:
\begin{equation*}
\begin{cases}
V_{21}=|V_{12}| e^{i \phi_{21}} = |V_{12}| e^{-i \phi_{12}}\\
V_{12}=|V_{12}| e^{i \phi_{12}}
\end{cases}
\end{equation*} We have to find phases $e^{i\alpha_1}$ and $e^{i\alpha_2}$. Complex numbers are equal when their phases and modules are respectively equal. I have no problem with finding the modules. However, phases are a completely different thing.
So for phases we have:

\begin{equation*}
\begin{cases}
e^{i\alpha_1} = e^{i\pi} e^{i\phi_{21}}e^{i\alpha_2}\\
e^{i\pi} e^{i\phi_{12}}e^{i\alpha_1} = e^{i\alpha_2}
\end{cases}
\end{equation*}

Which can be written as:
\begin{equation*}
\begin{cases}
e^{i(\alpha_1 - \alpha_2)} = e^{i\pi} e^{i\phi_{21}}\\
e^{i(\alpha_1 - \alpha_2)} = e^{-i\pi} e^{-i\phi_{12}} = e^{-i\pi} e^{i\phi_{21}}
\end{cases}
\end{equation*}

Multiplying by each side:
\begin{equation*}
e^{i2(\alpha_1 - \alpha_2)} = e^{i2\phi_{21}}
\end{equation*}
\begin{equation*}
e^{i(\alpha_1 - \alpha_2)} = e^{i\phi_{21}} = \frac{V_{21}}{|V_{12}|}
\end{equation*}

I don't know how to find the phases $e^{i\alpha_1}$ and $e^{i\alpha_2}$. I can see from the solution that $e^{i\alpha 1} = \frac{V_{12}}{|V_{12}|}$ and $e^{i\alpha 2}=\frac{V_{21}}{|V_{12}|}$ but how to obtain it from the equations?

 

[NateD]

Any help is appreciated.The answer to your question lies in the equation you derived:$$e^{i(\alpha_1 - \alpha_2)} = \frac{V_{21}}{|V_{12}|}$$This equation tells us that the difference between the phases is equal to the ratio of $V_{21}$ to $|V_{12}|$. Now, if we know one of these phases, we can use this equation to calculate the other.Let's say we know $\alpha_1$. Then we can calculate $\alpha_2$ using the equation above:$$\alpha_2 = \alpha_1 - \phi_{21}$$where $\phi_{21}$ is the phase of $V_{21}$. Similarly, if we know $\alpha_2$, then we can calculate $\alpha_1$ using the equation:$$\alpha_1 = \alpha_2 + \phi_{21}$$Once we know the phases, we can calculate the values of $e^{i\alpha_1}$ and $e^{i\alpha_2}$:$$e^{i\alpha_1} = e^{i\alpha_2 + \phi_{21}}$$$$e^{i\alpha_2} = e^{i\alpha_1 - \phi_{21}}$$