- #1
Janiceleong26
- 276
- 4
Q6c)
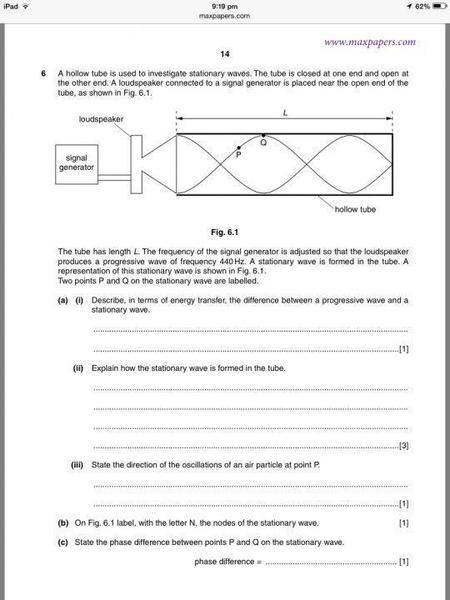
Why is the phase difference between two points in a stationary wave equals to zero?
I understand that a stationary wave is formed by two progressive waves which have the same amplitude, frequency, wavelength and speed, but traveling in opposite directions.
Why is the phase difference between two points in a stationary wave equals to zero?
I understand that a stationary wave is formed by two progressive waves which have the same amplitude, frequency, wavelength and speed, but traveling in opposite directions.