Theo2907
- 1
- 4
- TL;DR Summary
- Encountering strange results in dark counts of APD. Suspect reflections during APD dead time causing interference. Seeking quick insights to mitigate this issue. Any advice is appreciated.
Hey fellow researchers,
I'm currently working on an autocorrelation g(2) measurement setup using two Avalanche Photodiodes (APDs) connected through a multimode GRIN 50:50 beam splitter. Photons are delivered to the system via a multimode fiber (a PC-PC coupling to the beam splitter). However, I've been encountering a challenge and I believe it is related to the dead time of the APDs.
Below you can find a picture of the setup:
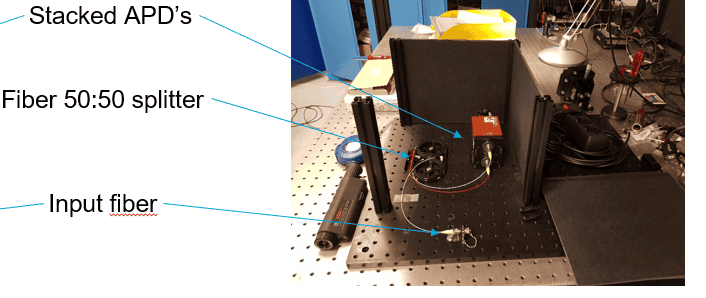
Before doing a complete g(2) measurement I wanted to check if my dark counts are incoherent (which should be the case). Unfortunately, I found that they are not incoherent, I did a measurement measuring the time difference between a measurement at APD 1 and APD 2 and I got peaks at around 20 ns and 80 ns.
You can see a screenshot of the graph below:
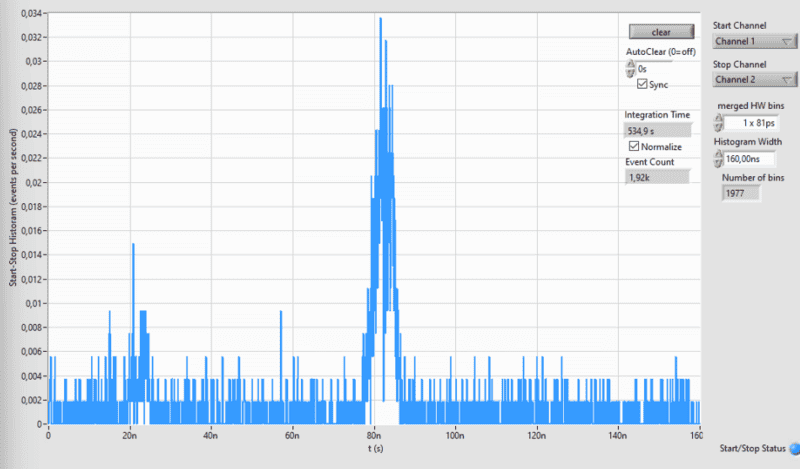
I couldn't explain it and no one in my lab has a good explanation for this phenomenon.
The peak at 20ns only happens when the beam splitter is actually coupled to the APDs, so a dark count measurement with the APDs not coupled to anything is completely incoherent (which is what we want).
The 80ns peak only happens when the multimode fiber(not present in the picture) is connected to the input fiber.
I do have a hypothesis though about this but I can't find any source online that verifies this.
I think that during the dead time of the APDs, incoming photons are being reflected, causing them to bounce back through the fiber. They are then reflected again at fiber couplings, the first one being the coupling between the input fiber and the MM fiber and there is another coupling from the MM fiber to another MM fiber.
Some people have advised me to use single-mode fibers instead and APC-APC couplings but if I use single-mode fiber my collection efficiency is not high enough and I don't get enough counts to make any measurement so that is not really an option. Later I want to measure light from single photon emitters in 4H-SiC so the collection efficiency is quite important.
I would greatly appreciate any insights, advice, or experiences you may have regarding this issue. Have you encountered similar challenges in your experiments? How did you address or mitigate the effects of this phenomenon?
Additionally, if anyone has recommendations for optimizing APD setups in autocorrelation measurements, please feel free to share. I'm open to suggestions and eager to learn from your expertise.
Thanks in advance for your help!
I'm currently working on an autocorrelation g(2) measurement setup using two Avalanche Photodiodes (APDs) connected through a multimode GRIN 50:50 beam splitter. Photons are delivered to the system via a multimode fiber (a PC-PC coupling to the beam splitter). However, I've been encountering a challenge and I believe it is related to the dead time of the APDs.
Below you can find a picture of the setup:
Before doing a complete g(2) measurement I wanted to check if my dark counts are incoherent (which should be the case). Unfortunately, I found that they are not incoherent, I did a measurement measuring the time difference between a measurement at APD 1 and APD 2 and I got peaks at around 20 ns and 80 ns.
You can see a screenshot of the graph below:
I couldn't explain it and no one in my lab has a good explanation for this phenomenon.
The peak at 20ns only happens when the beam splitter is actually coupled to the APDs, so a dark count measurement with the APDs not coupled to anything is completely incoherent (which is what we want).
The 80ns peak only happens when the multimode fiber(not present in the picture) is connected to the input fiber.
I do have a hypothesis though about this but I can't find any source online that verifies this.
I think that during the dead time of the APDs, incoming photons are being reflected, causing them to bounce back through the fiber. They are then reflected again at fiber couplings, the first one being the coupling between the input fiber and the MM fiber and there is another coupling from the MM fiber to another MM fiber.
Some people have advised me to use single-mode fibers instead and APC-APC couplings but if I use single-mode fiber my collection efficiency is not high enough and I don't get enough counts to make any measurement so that is not really an option. Later I want to measure light from single photon emitters in 4H-SiC so the collection efficiency is quite important.
I would greatly appreciate any insights, advice, or experiences you may have regarding this issue. Have you encountered similar challenges in your experiments? How did you address or mitigate the effects of this phenomenon?
Additionally, if anyone has recommendations for optimizing APD setups in autocorrelation measurements, please feel free to share. I'm open to suggestions and eager to learn from your expertise.
Thanks in advance for your help!