Physics - Archimedes principle

[bigpoppapump]

Im having trouble with the following question regarding Archimedes principle.

A wooden board with an area of 4.55m^2 is dropped into the dead sea (P sea- 1240 kg/m^-3). Calculate the proportion that would float above the surface. (P wood - 812 kg/m^-3).

My understanding is that the volume (V sub) of the submerged object over the total volume (V) of the object is equal to the density (P wood) of the Object over the density (P sea) of the water.

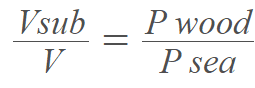
Because the question has given me an area and not a volume or even dimensions to calculate the volume, i am confused as to how to complete the question.

 

[skeeter]

$$\dfrac{4.5 \cdot d}{4.5 \cdot h} = \dfrac{812}{1240}$$
where $d$ is the submerged depth of the wood and $h$ is the physical height of the wood

note the question being asked is the proportion of the wood that floats above the surface …

$$\frac{h-d}{h} = 1-\frac{d}{h}
$$

 

[bigpoppapump]

Thank you. So by my calculations, this would have 35% floating above the surface.

 

[HOI]

The point is that you don't need to know the other dimensions- they cancel out of the fraction:
$\frac{V_{sub}}{V}= \frac{d_{sub}A}{dA}= \frac{d_{sub}}{d}$
where V is the volume of the stick, $V_{sub}$ is the volume of the submerged part, d is the thicknes of the stick, $d_{sub}$ is the thickness of the submerged part, and A is the cross section of the stick which is the same both submerged and not submerged.