Point charge in cavity of a spherical neutral conductor

[guyvsdcsniper]

Homework Statement

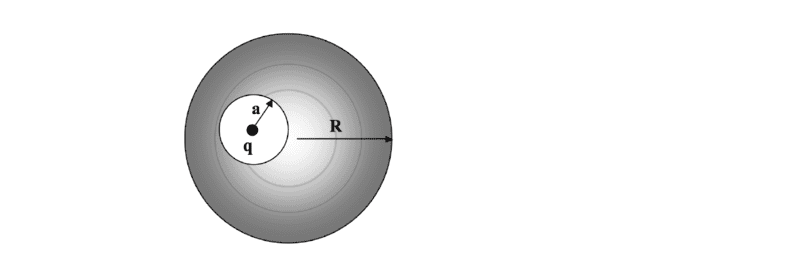
A spherical cavity (radius a) is dug out of a larger solid (neutral) conducting sphere of radius R,
see below. At the center of the cavity is a point charge q.
a)
If you move q a little off to one side, so it is no longer exactly at the center of the cavity, what changes? Please explain qualitatively and/or draw the resulting situation.

b)
Going back to the original problem (as shown), if you bring a new charge Q near the outside of the conductor,
which (if any) of your answers change? Please explain qualitatively and/or draw the resulting situation.

Relevant Equations

∫Eda=q/ϵ

For (a) this problem, the only thing I can see changing is the distribution of the negative charge on the inner wall of the cavity.

When the point charge is in the center of the cavity, you could say the induced charged is spread symmetrically on the inner cavity wall in order to oppose the point charge.

Well when we move the point charge a little off to one side, The induced charge would be more concentrated to where the the point charge is shifted to, making it lose its symmetry.

I believe the Electric Field would stay the same? If I drew a Gaussian Surface around the p.c., I should get the same E, there should still be the same #of flux passing through the surface, just one side having more lines than the other.For (b), only the outside of the conductor will be affected, mainly the distribution of the charges on the surface of the outer sphere. They will collect to repel whatever side the charge is brought near, similar to the previous problem.

The inside of the sphere, including the cavity, should be unaffected.

Am I correct?

 

[haruspex]

For a), you should also discuss the induced charge distribution on the outside surface of the sphere.

I believe the Electric Field would stay the same?

The field where?

Your argument that the rearrangement of outside surface charges should not affect the inside (and v.v.?) are a bit handwaving. What do you know about mirror image charges in respect of spheres?

 

[guyvsdcsniper]

For a), you should also discuss the induced charge distribution on the outside surface of the sphere.

Thats a good point for a, I didn't think about that. Would the charge distribution mimic that of the inner wall of the cavity?

The field where?

Your argument that the rearrangement of outside surface charges should not affect the inside (and v.v.?) are a bit handwaving. What do you know about mirror image charges in respect of spheres?

I was referring to the Electric Field of the point charge in the cavity. I don't believe shifting its position should effect its Electric Field.

I do not no much to be honest. Are you referring to method of images?

 

[haruspex]

Are you referring to method of images?

Yes.

Thats a good point for a, I didn't think about that. Would the charge distribution mimic that of the inner wall of the cavity?

Again, what you need is the method of images. If you put a point charge outside a grounded spherical conductor the induced charge is, as far as points outside the sphere are concerned, equivalent to what?
Can you see how that can be applied to the given case of a point charge inside this ungrounded conductor?

 

[guyvsdcsniper]

Yes.

Again, what you need is the method of images. If you put a point charge outside a grounded spherical conductor the induced charge is, as far as points outside the sphere are concerned, equivalent to what?
Can you see how that can be applied to the given case of a point charge inside this ungrounded conductor?

So I went over an example given in griffiths for that particular situation and barring the calculation of the potential, this is the sketch I got.

The induced charge on the neutral conductor is negative, with the charge density being concentrated on the side closest to the charge outside the conductor.

I am having a hard time seeing how that can be applied to the given case of a point charge inside this ungrounded conductor.

 

[haruspex]

So I went over an example given in griffiths for that particular situation and barring the calculation of the potential, this is the sketch I got.

The induced charge on the neutral conductor is negative, with the charge density being concentrated on the side closest to the charge outside the conductor.

View attachment 297761

I am having a hard time seeing how that can be applied to the given case of a point charge inside this ungrounded conductor.

Sorry, I think I have blundered. I thought the method of images applied to a grounded spherical shell shows that the distribution of charges on the sphere is equivalent, as far as the field outside the sphere is concerned, to a single opposite point charge at a certain position inside the sphere.

But I now believe that is wrong. It only arranges for a zero potential at the sphere. Anyway, the rest of my argument was as follows:

From this we can see that there is a way to distribute charges on a spherical shell which, as far as the field outside the sphere is concerned, is equivalent to a single point charge at a certain position inside the sphere. In particular, that point charge can be equal and opposite to an actual charge at any given position inside a shell.
So suppose that this is how the charges on the inside of the cavity will distribute. Having done so, there is no net field outside the cavity from the point charge and the surface charge. To complete the picture we need a charge distribution on the outside of this sphere such that:
1. There is no net field in the solid region of the sphere, and
2. There is no net charge on the sphere?

 

[guyvsdcsniper]

Sorry, I think I have blundered. I thought the method of images applied to a grounded spherical shell shows that the distribution of charges on the sphere is equivalent, as far as the field outside the sphere is concerned, to a single opposite point charge at a certain position inside the sphere.

But I now believe that is wrong. It only arranges for a zero potential at the sphere. Anyway, the rest of my argument was as follows:

From this we can see that there is a way to distribute charges on a spherical shell which, as far as the field outside the sphere is concerned, is equivalent to a single point charge at a certain position inside the sphere. In particular, that point charge can be equal and opposite to an actual charge at any given position inside a shell.
So suppose that this is how the charges on the inside of the cavity will distribute. Having done so, there is no net field outside the cavity from the point charge and the surface charge. To complete the picture we need a charge distribution on the outside of this sphere such that:
1. There is no net field in the solid region of the sphere, and
2. There is no net charge on the sphere?

Well there would have to be a charge that is opposite in sign to the induced charge on the cavity wall.

But there would be a +q on the outside of the sphere even if the the +q and the induced charge in the cavity cancel each other.

I guess the question is how that +q on the outside of the sphere distributes. I can't see why it wouldn't be evenly distributed?

 

[SammyS]

Well there would have to be a charge that is opposite in sign to the induced charge on the cavity wall.

But there would be a +q on the outside of the sphere even if the the +q and the induced charge in the cavity cancel each other.

I guess the question is how that +q on the outside of the sphere distributes. I can't see why it wouldn't be evenly distributed?

For question a):
Yes the +q charge on the outside of sphere is evenly distributed.

 

[vela]

I believe the Electric Field would stay the same? If I drew a Gaussian Surface around the p.c., I should get the same E, there should still be the same #of flux passing through the surface, just one side having more lines than the other.

The electric field is a vector field. When you say it doesn't change, you're saying that at every point in space, the vector assigned to that point is the same before and after. I don't think that's what you mean.

You noted while the total flux through the gaussian surface is unchanged, there will be more field lines on one side than the other. Remember the density of field lines is greater where the electric field has greater magnitude.