- #1
bonodut
- 8
- 0
I was taught that when you have a polynomial fraction where the denominator is of a higher degree than the numerator, it can't be reduced any further. This seems wrong to me for a couple of reasons.
1. If the denominator can be factored some of the terms may cancel out
2. Say you have the expressions
a)
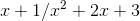
b)
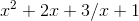
B divides out to
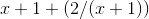
Couldn't A be expressed as the reciprocal of this? If so, which is the simplest form? A or this reciprocal? Why?
I don't think I understand what "simplest form" means in terms of polynomial fractions. Hopefully this question makes sense.
1. If the denominator can be factored some of the terms may cancel out
2. Say you have the expressions
a)
b)
B divides out to
Couldn't A be expressed as the reciprocal of this? If so, which is the simplest form? A or this reciprocal? Why?
I don't think I understand what "simplest form" means in terms of polynomial fractions. Hopefully this question makes sense.