- #1
Qshadow
- 27
- 0
I am interested in theoretical (what if) possibility of our Earth having more than one moon,
I wonder if they can be arranged at the same orbital resonance as the Galilean moons 1:2:4
If yes, would such system be stable?
If yes, should our Moon be the first, second or third satellite out of the three for the system to be most stable?
Assuming such system is possible and stable,
I have made some calculations based on the assumption that our Moon is second (Moon2), and using the Kepler's Third Law, for calculating the distance of all the moons as function of the orbital period T (see Orbital Period wiki):
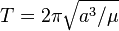
Moon1 distance: (((27.3*24*60*60)^2*398600)/(16*3.14^2))^(1/3) = 241,000 Km
Moon2 distance: (((27.3*24*60*60)^2*398600)/(4*3.14^2))^(1/3) = 383,000 Km
Moon3 distance: (((27.3*24*60*60)^2*398600)/(1*3.14^2))^(1/3) = 608,000 Km
is this correct way to do this, or am i missing something, and this is not the way to calculate this distances?
If no, please point me to correct info,
Thanks,
Qshadow.
I wonder if they can be arranged at the same orbital resonance as the Galilean moons 1:2:4
If yes, would such system be stable?
If yes, should our Moon be the first, second or third satellite out of the three for the system to be most stable?
Assuming such system is possible and stable,
I have made some calculations based on the assumption that our Moon is second (Moon2), and using the Kepler's Third Law, for calculating the distance of all the moons as function of the orbital period T (see Orbital Period wiki):
Moon1 distance: (((27.3*24*60*60)^2*398600)/(16*3.14^2))^(1/3) = 241,000 Km
Moon2 distance: (((27.3*24*60*60)^2*398600)/(4*3.14^2))^(1/3) = 383,000 Km
Moon3 distance: (((27.3*24*60*60)^2*398600)/(1*3.14^2))^(1/3) = 608,000 Km
is this correct way to do this, or am i missing something, and this is not the way to calculate this distances?
If no, please point me to correct info,
Thanks,
Qshadow.