Potential at centre of cylinder

[Saitama]

Homework Statement

Homework Equations

The Attempt at a Solution

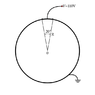
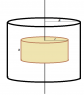
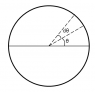
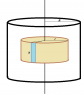
I have no idea on this one. I can't understand what happens when some portion of the cylinder is earthed and other is maintained at 110V. The potential of the earthed portion is 0V but how does that even help me?

Any help is appreciated. Thanks!

 

[voko]

Is this not just a simple application of the superposition of electric potentials?

 

[haruspex]

Is this not just a simple application of the superposition of electric potentials?

Don't you need to know the charge distributions to do that? The OP is a Dirichlet boundary value problem, no?

 

[Saitama]

Don't you need to know the charge distributions to do that? The OP is a Dirichlet boundary value problem, no?

What is this Dirichlet boundary value problem?

 

[TSny]

Suppose you choose an imaginary circle of radius r < R lying in a plane perpendicular to the axis of the cylinder and centered on the axis of the cylinder.

Try to show that the integral ∫₀^2π V(r,θ)dθ is independent of the value of r, where the integral is over the circle of radius r and θ is the polar angle.

If you can do that, then you can relate V on the axis to the values of V on the cylinder.

 

[Saitama]

Suppose you choose an imaginary circle of radius r < R lying in a plane perpendicular to the axis of the cylinder and centered on the axis of the cylinder.

Try to show that the integral ∫₀^2π V(r,θ)dθ is independent of the value of r, where the integral is over the circle of radius r and θ is the polar angle.

If you can do that, then you can relate V at the center to the values of V on the cylinder.

I understand what you say here but how am I even supposed to find $V(r,\theta)$?

I don't even have the charge distribution, only the potential of two portions of cylinder.

 

[TSny]

You don't need to find V(r, θ) in order to show that ∫V(r,θ)dθ is independent of r.

Although not obvious, you can show this by starting with Gauss's law for a closed cylindrical Gaussian surface of radius r as shown. See if you can express the net flux through the surface in terms of an integral of E_r, the radial component of the electric field. Then relate E_r to V(r,θ) inside the integral.

 

[Saitama]

Although not obvious, you can show this by starting with Gauss's law for a closed cylindrical Gaussian surface of radius r as shown. See if you can express the net flux through the surface in terms of an integral of E_r, the radial component of the electric field. Then relate E_r to V(r,θ) inside the integral.

Flux through the Gaussian surface is $\phi=E_r\cdot 2\pi rh$ but this does not involve any integral.

I think I cannot consider $E_r$ as constant over the whole Gaussian surface, right?

From Gauss law, $\phi=\int \vec{E_r}\cdot \vec{dA}$. $\displaystyle E_r=-\frac{dV}{dr}$ but this does not involve $\theta$.

 

[TSny]

Flux through the Gaussian surface is $\phi=E_r\cdot 2\pi rh$ but this does not involve any integral.

E_r cannot be assumed to be constant over the surface. So, can you bring it out of the flux integral?

[EDIT]

$E_r=-\frac{dV}{dr}$ but this does not involve $\theta$.

Since V is a function of r and θ, ∂V/∂r will also be a function of r and θ.

 

[Saitama]

Since V is a function of r and θ, ∂V/∂r will also be a function of r and θ.

I don't know how to solve $dV(r,\theta)/dr$.

 

[TSny]

You don't need to solve dV/dr. Express the flux integral in terms of ∂V/∂r. The goal is to use the flux integral to show d/dr ∫V(r,θ)dθ = 0, which means ∫V(r,θ)dθ over a circle of radius r is a constant (independent of the size of the circle).

 

[haruspex]

What is this Dirichlet boundary value problem?

See http://physics.usask.ca/~hirose/p812/notes/Ch2.pdf.

If the potential is specified on a closed surface, the potential off the surface is uniquely determined in terms of the surface potential. This is known as Dirichlet's boundary value problem
​

 

[Saitama]

You don't need to solve dV/dr. Express the flux integral in terms of ∂V/∂r. The goal is to use the flux integral to show d/dr ∫V(r,θ)dθ = 0, which means ∫V(r,θ)dθ over a circle of radius r is a constant (independent of the size of the circle).

Do I need to simply substitute $E_r=∂V/∂r$?

See http://physics.usask.ca/~hirose/p812/notes/Ch2.pdf.

That's too much mathematics. Is there no easier way to this problem?

 

[voko]

Don't you need to know the charge distributions to do that? The OP is a Dirichlet boundary value problem, no?

Well, the solution to a Dirichlet problem, involving Green's function, is an application of superposition :)

But you are correct in that it is not simple and not direct as my original suggestion implied. I think I misunderstood the problem initially.

 

[voko]

That's too much mathematics. Is there no easier way to this problem?

This is the standard approach to such problems. Besides, most of mathematics there is not for you do, but merely to follow. If you follow, you will see in one example that most of what needs to be done for this problem is done there, you just need to plug in your data to get the solution.

 

[TSny]

Do I need to simply substitute $E_r=∂V/∂r$?

Yes. What does Gauss' law look like for the chosen Gaussian surface after making the substitution $E_r=-∂V/∂r$.

That should bring you to within a few simple steps of the answer to the question.

 

[Saitama]

Yes. What does Gauss' law look like for the chosen Gaussian surface after making the substitution $E_r=-∂V/∂r$.

That should bring you to within a few simple steps of the answer to the question.

$\displaystyle \phi=\int -\frac{∂V}{∂r}dA$

But what is $dA$?

 

[TSny]

$\displaystyle \phi=\int -\frac{∂V}{∂r}dA$

But what is $dA$?

What part of the Gaussian surface are you integrating over here?

 

[Saitama]

What part of the Gaussian surface are you integrating over here?

Is $dA=rd\theta dy$ where y is the distance of the patch(dA) from the top base of the Gaussian surface?

 

[haruspex]

That's too much mathematics. Is there no easier way to this problem?

Section 3.1.2 of http://teacher.pas.rochester.edu/PHY217/LectureNotes/Chapter3/LectureNotesChapter3.pdf is more accessible, but it merely quotes the result you need (Property 2), doesn't prove it.

 

[Saitama]

Section 3.1.2 of http://teacher.pas.rochester.edu/PHY217/LectureNotes/Chapter3/LectureNotesChapter3.pdf is more accessible, but it merely quotes the result you need (Property 2), doesn't prove it.

V has no local maxima or minima; all extremes occur at the boundaries

How do I get my answer from this statement? The potential at the boundaries is given, I don't see how can I deduce the potential at axis from this property. :(

 

[TSny]

Is $dA=rd\theta dy$ where y is the distance of the patch(dA) from the top base of the Gaussian surface?

Ok. But you can just use vertical strips of height h.

 

[Saitama]

Ok. But you can just use vertical strips of height h.

But still I don't know how to solve it after substituting $dA=rhd\theta$.

 

[TSny]

Substitute your expression for dA into your flux integral expressed in terms of ∂V/∂r. Remember Gauss told you what the flux must equal.

 

[haruspex]

How do I get my answer from this statement? The potential at the boundaries is given, I don't see how can I deduce the potential at axis from this property. :(

Sorry, I should have written Property 1.

 

[Saitama]

Sorry, I should have written Property 1.

Property 1 states that "The value of V at a point (x, y) is equal to the average value of V around this point...", does this mean $V_{axis}$ is the average of 110 V and 0 V, i.e 55.5 V?

Substitute your expression for dA into your flux integral expressed in terms of ∂V/∂r. Remember Gauss told you what the flux must equal.

The flux is zero i.e
$$\int -\frac{∂V}{∂r}rhd\theta=0$$
But what am I supposed to do with this?

 

[voko]

Property 1 states that "The value of V at a point (x, y) is equal to the average value of V around this point...", does this mean $V_{axis}$ is the average of 110 V and 0 V, i.e 55.5 V?

Of course not. Which should be quite obvious to you, provided you read what Property 1 states ENTIRELY.

 

[Saitama]

Of course not. Which should be quite obvious to you, provided you read what Property 1 states ENTIRELY.

Property 1 involves a surface integral. Can I take V out of the integral?

I don't know how to evaluate surface integrals. :(

 

[voko]

Property 1 involves a surface integral. Can I take V out of the integral?

I don't know how to evaluate surface integrals. :(

Property 1 of 3.1.2 does not involve any surface integrals. Please do read carefully what you are given.

 

[Saitama]

Property 1 of 3.1.2 does not involve any surface integrals. Please do read carefully what you are given.

Woops, I used the wrong term I think. Whatever that integral is called, I don't have any idea about evaluating it. :(

Can I take V out of the integral?