Potential energy between two oppositely charged ions in a crystal

[geoffreythelm]

Hi, I am having trouble with the following:

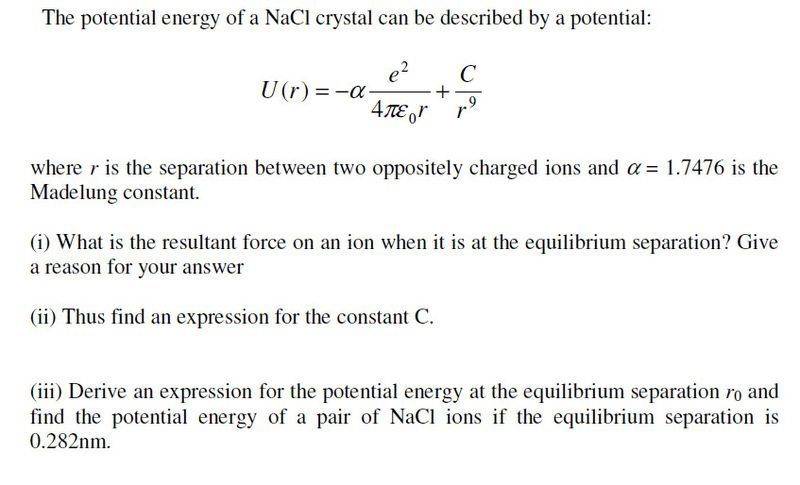
All I have really done is differentiate the function to give an expression for F(r), but I am a bit clueless about the rest. How can there be equilibrium if the two ions are attracted to each other?

Cheers,
GeoffreyThelm

 

[kuruman]

Your potential has two terms, are they both attractive?

 

[geoffreythelm]

I see.. So one (the first term?) is due to the attractive force from one ion, and vice versa for the other? So c is going to end up being something which looks like electric potential should. So is the point of equilibrium the point between the ions where the first term= the second term?

 

[kuruman]

The point of equilibrium is where the sum of the forces is zero. When you have a potential, it is where dU/dx = 0, i.e. where the potential has an extremum (maximum or minimum). In this case it had better be a minimum. Consider plotting this potential to see what it looks like.