Potential energy of an hemispheric shell

[BSJ90]

Homework Statement

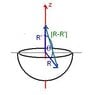
Given that the hemisphere has a charge +Q distributed through its surface with radius a. Find the electric potential on any point on z axis (the plane of the hemisphere is oriented in positive z direction).

Homework Equations

\phi = \int\frac{kQ}{|R-R'|}*ds
(surface integral)

The Attempt at a Solution

So i decided to break this problem into little pieces of the surface using some trig.

I made a angle θ from the plane of the hemisphere around the cross section (so there is semi circles) and then an angle \varphi on the xy-plane.

I found ds to be a^{2}d\varphid\theta (using the arc lengths of my angles and radii).

Now my problem is finding an equation for the vector R-R'. I tried making it a function of \theta (i.e. i got (sqrt(z^{2} + a^{2}sin^{2}(\theta)
but the integral was not a nice one and it lead me to believe that it wasn't correct. if someone can help me along that would be great!

 

[ehild]

Is the charge evenly distributed on the surface?

Make a picture and indicate R and R'!


ehild

 

[BSJ90]

oh yeah evenly distributed on surface. I made a paint object to help clarify. R' is to the ds and R is to the point on the z axis. I know R has to be z but I am having a problem figuring out R'. Thanks for this and the last problem you help me with.

 

[ehild]

Nice picture! You need the magnitude of the vector difference of R-R'. What about using the Law of Cosines?
To make your task more clear I draw an other picture with Paint (using the option to draw circle, ellipse, straight line )

ehild

 

[BSJ90]

ok so should my theta be from the z axis and i think that changes my whole set up oh wait i can use 90 + theta and it would work. Ok thanks time to go to work on it. Plus i only really care about the z direction.

 

[BSJ90]

I get my |R- R'| = \sqrt{ a^2 +z^2 + 2azsin(θ)}. look right? Umm this isn't turning out to be a easy integral. I am thinking mabye go back to Cartesian.

 

[ehild]

The official way is to count theta from the positive x axis. Take care of the surface element: it is R² sin(θ) dθ dφ with the usual way. See:

http://hyperphysics.phy-astr.gsu.edu/hbase/sphc.html

If you stick to your theta, find it out.

ehild

 

[BSJ90]

I just need to add 90 degrees to my theta so it restricts it to my hemisphere not a whole sphere. The ds stays the same except for that sin(θ) term which i added.

 

[ehild]

It will not be sin(theta) in the surface element of your special spherical system of coordinates.

ehild