Power dissipated in this circuit with 2 batteries and 3 resistors

[patric44]

Homework Statement

Calculate the power dissipated in the total circuit

Relevant Equations

P = I^2R

Hi guys
I am struggling to find the power dissipated in the total circuit , my answer didn't match the solution in the book

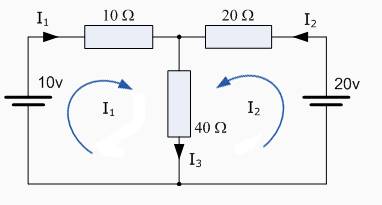
the circuit is simple i used Kirchhoffs rules to find the following :
loop 1 :
$$10-10I_{1}-40I_{3} = 0$$
loop 2:
$$20-20I_{2}-40I_{3} = 0$$
and $$ I_{1}+I_{2}=I_{3}$$
the current through each resistor was
I1 = -0.142857A
I2 = 0.42857A
I3 = 0.2857A
and the the power dissipated in the total circuit is
$$((I1^2)*R1)+((I2^2)*R2)+((I3^2)*R3) = 7.1428W$$
but the books answer is 8.57W
am I doing something wrong ?! or the book is incorrect

 

[kuruman]

My answer agrees with yours.

 

[Gavran]

A circuit element generates energy when the current leaves the element through the higher voltage terminal (+) and enters the element through the lower voltage terminal (-). ($P<0~W$)
A circuit element dissipates energy when the current enters the element through the higher voltage terminal (+) and leaves the element through the lower voltage terminal (-). ($P>0~W$)

$P_{1}=0,20~W$ - the power at the $10~\Omega$ resistor
$P_{2}=3,67~W$ - the power at the $20~\Omega$ resistor
$P_{3}=3,27~W$ - the power at the $40~\Omega$ resistor
$P_{4}=1,43~W$ - the power at the $10~V$ battery

$P = P_{1}+ P_{2}+ P_{3}+ P_{4} = 0,20~W + 3,67~ W + 3,27~ W + 1,43~ W = 8,57~ W$ - the power dissipated in the total circuit

The book is correct.

 

[DaveE]

It's kind of a trick question. You are correct if the batteries are real things. Then then the current I₁ will charge the 10V battery and is stored, not dissipated. But if that is interpreted as an ideal voltage source, then that power is dissipated and the book is correct. IMO, they should have used a voltage source symbol in the schematic to avoid confusion, or otherwise explained things better.

 

[erobz]

It's kind of a trick question. You are correct if the batteries are real things. Then then the current I₁ will charge the 10V battery and is stored, not dissipated. But if that is interpreted as an ideal voltage source, then that power is dissipated and the book is correct. IMO, they should have used a voltage source symbol in the schematic to avoid confusion, or otherwise explained things better.

If the battery is being charged then the circuit is not at steady state? These problems are in done in introductory circuits, but "how can they be" is now the question I have.

I guess you are saying the book intends the ideal voltage source which cannot store the energy, thus it must be "lost" across the ideal voltage source. I must have been sick the day they discussed the effects of "real" vs "ideal" voltage sources.

 

[berkeman]

A circuit element generates energy when the current leaves the element through the higher voltage terminal (+) and enters the element through the lower voltage terminal (-). ($P<0~W$)
A circuit element dissipates energy when the current enters the element through the higher voltage terminal (+) and leaves the element through the lower voltage terminal (-). ($P>0~W$)

$P_{1}=0,20~W$ - the power at the $10~\Omega$ resistor
$P_{2}=3,67~W$ - the power at the $20~\Omega$ resistor
$P_{3}=3,27~W$ - the power at the $40~\Omega$ resistor
$P_{4}=1,43~W$ - the power at the $10~V$ battery

$P = P_{1}+ P_{2}+ P_{3}+ P_{4} = 0,20~W + 3,67~ W + 3,27~ W + 1,43~ W = 8,57~ W$ - the power dissipated in the total circuit

The book is correct.

Welcome to PF.

This homework question thread is old enough that it's okay to post answers to the question. But in general, we do not allow anyone to post answers to homework threads except the Original Poster (the student). Here at PF the student must do the bulk of the work on their homework assignments.

 

[TonyStewart]

The book is correct.
Remember that all voltage sources that supply power are negative unless they are absorbing charge current as in this case for I1.
So all the power comes from I2 so the assumed flow direction is +ve current which means going into the negative terminal or P= I2*(-V2) =0.42857A *(-20) = -8.5714 W.

By convention, we assume generated or supplied power is -ve and resistive loads dissipate heat which is +ve power. If we said we are supplying negative watts that is wrong like a "double negative".

You might think, so can does an ideal voltage source absorb power without heating up. In reality is a very large capacitor, infinite C in theory, or some C being recharged , with ESR = Rs, where real sources will heat up being charged with some % efficiency ratio. Ideal sources are thus lossless infinite Capacitors.

 

[Steve4Physics]

My tuppence worth…

In the context of simple electrical circuits ‘dissipation’ invariably refers to ohmic heating – the production of ‘heat’ when a current passes through some resistance.

The original (2+ year old) question shows 2 ideal voltage sources. (They are referred to as ‘batteries’ but are represented in the diagram as cells.) By implication, they are ideal so have no internal resistance and do not contribute to the dissipation. Lossless conversion between ‘chemical energy’ and ‘electrical energy’ is not dissipation.

IMO we only need to consider the power dissipated in the three resistors.

 

[TonyStewart]

My tuppence worth…

In the context of simple electrical circuits ‘dissipation’ invariably refers to ohmic heating – the production of ‘heat’ when a current passes through some resistance.

The original (2+ year old) question shows 2 ideal voltage sources. (They are referred to as ‘batteries’ but are represented in the diagram as cells.) By implication, they are ideal so have no internal resistance and do not contribute to the dissipation. Lossless conversion between ‘chemical energy’ and ‘electrical energy’ is not dissipation.

IMO we only need to consider the power dissipated in the three resistors.

Nice try but Kirchoff rules apply to add up all the power in the loop to support laws of conservation that the net power must be 0.

$0 = P_{1}+ P_{2}+ P_{3}+ P_{4}+ P_{5}$ where P_5 is the battery providing all the power with the highest voltage.
$P_{5} = P_{1}+ P_{2}+ P_{3}+ P_{4}$

 

[vanhees71]

The book is correct.
Remember that all voltage sources that supply power are negative unless they are absorbing charge current as in this case for I1.
So all the power comes from I2 so the assumed flow direction is +ve current which means going into the negative terminal or P= I2*(-V2) =0.42857A *(-20) = -8.5714 W.

By convention, we assume generated or supplied power is -ve and resistive loads dissipate heat which is +ve power. If we said we are supplying negative watts that is wrong like a "double negative".

You might think, so can does an ideal voltage source absorb power without heating up. In reality is a very large capacitor, infinite C in theory, or some C being recharged , with ESR = Rs, where real sources will heat up being charged with some % efficiency ratio. Ideal sources are thus lossless infinite Capacitors.

But this is not dissipated but rather stored in the other battery!

 

[DaveE]

If the battery is being charged then the circuit is not at steady state?

It's not. But they never said it was a steady state problem. Also, these idealized networks are really only used in practice to construct simplified models of real stuff. If the batteries are really big, then a constant voltage is a good approximation. Then it behaves just like a steady state model. "Steady state" is always a relative term IRL; these circuits weren't built at the dawn of creation.

Again, it depends on the semantics. Is that a battery or a voltage source? The only real difference appears when they used the word "dissipate".

 

[Steve4Physics]

Nice try but Kirchoff rules apply to add up all the power in the loop to support laws of conservation that the net power must be 0.

$0 = P_{1}+ P_{2}+ P_{3}+ P_{4}+ P_{5}$ where P_5 is the battery providing all the power with the highest voltage.
$P_{5} = P_{1}+ P_{2}+ P_{3}+ P_{4}$

The issue is the correct use of terminology. ‘Dissipation’, if used correctly here, specifically means heating. The only components which get heated are the three resistors. The power dissipated is $P_1 + P_2 +P_3$.

Kirchhoff's (check spelling by the way!) voltage law follows from the law of conservation of energy. In terms of power it tells us $P_1+ P_2 +P_3+P_4+P_5 = 0$.

It follows that dissipated power $= -(P_4+P_5)$. This is the rate of heat production in the circuit.

 

[nasu]

The book is correct.
Remember that all voltage sources that supply power are negative unless they are absorbing charge current as in this case for I1.
So all the power comes from I2 so the assumed flow direction is +ve current which means going into the negative terminal or P= I2*(-V2) =0.42857A *(-20) = -8.5714 W.

By convention, we assume generated or supplied power is -ve and resistive loads dissipate heat which is +ve power. If we said we are supplying negative watts that is wrong like a "double negative".

You might think, so can does an ideal voltage source absorb power without heating up. In reality is a very large capacitor, infinite C in theory, or some C being recharged , with ESR = Rs, where real sources will heat up being charged with some % efficiency ratio. Ideal sources are thus lossless infinite Capacitors.

You are just changing the usual meaning of "dissipated" to fit the answer in the book. Are you the author of that book?

 

[TonyStewart]

You are just changing the usual meaning of "dissipated" to fit the answer in the book. Are you the author of that book?

Where? No I am not. Dissipated only refers to real power lost in heat. read #12
Reactive elements "absorb" power over time storing energy (L,C)
Voltage sources are ideal infinite capacitors and thus may charge or discharge.

 

[nasu]

Not all that power is "dissipated". Some goes into "charging" the 10V battery. Assuming it is rechargeable. In general, not all the power provided by a source is dissipated, in the sense of converted to thermal energy which is eventually transfered to the environment. Here the 8.57 W is the power "provided" by the 20 V battery. The power lost in the resistors is clearly "dissipated" by the usual meaning used in this context. The problem is about the rest of the power, associated with the 10 V battery. It would be a stretch to call it "dissipated" if the battery is assumed ideal. On the other hand, if it's not a rechargeable battery, it is not stored in the battery either. Something bad will happen to a real, non-rechargeable, battery connected like this. Maybe we call this "dissipation" as well.

 

[TonyStewart]

You seem to think that I do not agree with your 1st statement. Some power is absorbed ( charged) in V1 while the source is generated, or discharged from V2. Neither of these are considered heat. ( consider same as infinite lossless capacitor)

But it may be you who does not understand what I wrote. No, we do not call absorption (or charge) ="dissipation" That only occurs in real parts with ESR.

 

[nasu]

Then how do you figure that the book answer is correct? The power dissipated in the resistors is 7.1 W and the book seems to say that is 8.6 W. What did I missunderstand?

 

[haruspex]

Dissipated only refers to real power lost in heat.

Ok, so the three resistors dissipate, while the 20v battery generates and the (perfectly rechargeable) 10v battery absorbs. Total dissipated = 7.14W = generated - absorbed = 8.57W - 1.43W.
The book is wrong.

 

[DaveE]

You might think, so can does an ideal voltage source absorb power without heating up. In reality is a very large capacitor, infinite C in theory, or some C being recharged , with ESR = Rs, where real sources will heat up being charged with some % efficiency ratio. Ideal sources are thus lossless infinite Capacitors.

That's an odd definition of an ideal voltage source. The normal one is $v(t) = V_o$ with $V_o$ a given constant. But I suppose your definition of $v(t) = \lim_{C \rightarrow +\infty} [{(\frac{1}{C})\int_0^t i(\tau) \, d \tau + v(0)}]$ may also work. IDK, it seems OK. I'd have to think about it, and I don't see a good reason to do that.

PS: Nope, couldn't not think about it. This is just wrong. For any value of C, even really, really big ones (arbitrarily big) this behaves as a capacitor and the voltage changes accordingly, perhaps an arbitrarily small amount. No, I'm not going to do the $\delta - \Sigma$ proof, I'd probably do it slightly wrong and derail an already too long thread. There are batteries, capacitors, and voltage sources. They all behave differently. They all have different schematic symbols. The OP's HW was mistaken in using the battery symbol and assuming energy was dissipated, for their answer they should have used a voltage source symbol.

 

[vanhees71]

Where? No I am not. Dissipated only refers to real power lost in heat. read #12
Reactive elements "absorb" power over time storing energy (L,C)
Voltage sources are ideal infinite capacitors and thus may charge or discharge.

Yes, indeed, and in a capacitor no energy is dissipated but it's stored in the electric field. In an ideal coil the energy is also not dissipated but stored in the magnetic field. Dissipation refers to heat production and nothing else (at least in the meaning of the word in standard physics, which is what's discussed in this forum).

A battery is of course far from being a simple capacitor. There you have also chemistry involved. Strictly speaking they provide not "voltage" but an "electromotive force".

 

[TonyStewart]

The issue is the correct use of terminology. ‘Dissipation’, if used correctly here, specifically means heating. The only components which get heated are the three resistors. The power dissipated is $P_1 + P_2 +P_3$.

Kirchhoff's (check spelling by the way!) voltage law follows from the law of conservation of energy. In terms of power it tells us $P_1+ P_2 +P_3+P_4+P_5 = 0$.

It follows that dissipated power $= -(P_4+P_5)$. This is the rate of heat production in the circuit.

I agree. It is a misleading question and I change my opinion now and agree the book used the wrong choice of words but the intent was OK. The question should have said instead, "HOW MUCH NET POWER IS TRANSFERRED?

$P_1+ P_2 +P_3+P_4+P_5 = 0$ Kirchhoff's Law prevails and put positive values on each side of the equation to show source and loads.

 

[TonyStewart]

That's an odd definition of an ideal voltage source. The normal one is $v(t) = V_o$ with $V_o$ a given

Agreed, it is my "odd definition" to say an ideal Voltage source is just an ideal charged infinite Capacitor while both this and an ideal voltage source never sag, and both can transfer energy in or out without loss.

But if you think about batteries as voltage sources, they do have an equivalent Capacitance with the change of energy states above the knee voltage threshold of the battery.

An 18650 battery is about 10k Farads fully charged and may look like a poor lossy Supercap with high ESR at low %SoC or State of Charge levels. Also the Double-Electric Layer effects means there is more than one RC equivalent network in a battery giving it a short-term memory effect after a pulsed short.

All voltage sources have some impedance and in reality these are modelled with ESR which determines load regulation error. Then a range of caps each with an SRF and ESR and C value spreads the bandwidth for a low ESR(f) with some parasitic inductance < 1nH/mm that determines the Self Resonant Frequency (s) (SRF).

If I am wrong, I can be convinced and will admit it. Can others?

 

[nasu]

If I am wrong, I can be convinced and will admit it. Can others?

No, they cannot. It's just the two of us.