hello478
- 165
- 14
- Homework Statement
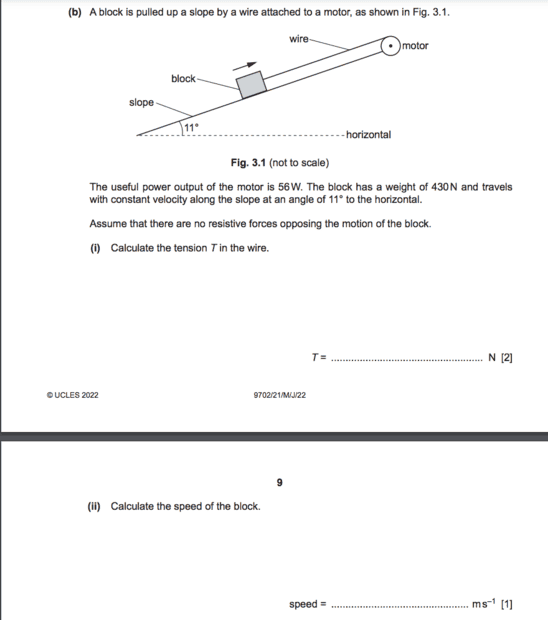
- in finding the speed, do we use the tension force or the weight?
and what is f, wouldnt it be zero? as resultant force is zero
what does f in this equation mean?
- Relevant Equations
- p=fv
my attempt: i solved it all correct but i dont understand a few things mentioned above...
82.04 * v = 56
so i got v as 0.68 m/s which is correct
but i dont understand the concept...
82.04 * v = 56
so i got v as 0.68 m/s which is correct
but i dont understand the concept...
