Pressure at bottom of three differently shaped cylinders

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

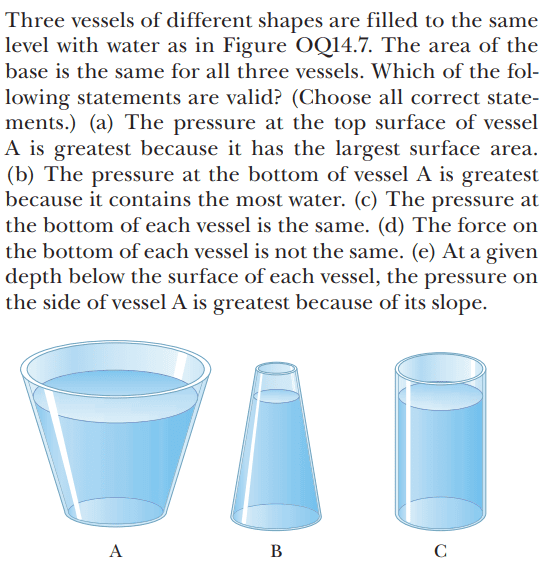
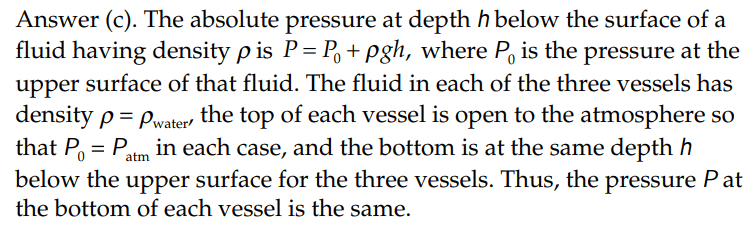
The solution is,

However, I thought the answer would be (b) and (e). I choose (b) because from the formula $P = \frac{F}{A}$ then since the vessel A has more water, then the water will have a larger weight $W_A > W_C > W_B$, therefore from$P = \frac{mg}{A}$ we conclude that since the area is the same for each vessel then $P_A > P_C > P_B$.

I choose (e) because I thought the pressure on the sides of A would be greatest since from each value of $h$ below the water, there is more water above in A since it circumference is largest at the top and decreases.

Could someone please why my answers is not correct?

Many thanks!

 

[jbriggs444]

However, I thought the answer would be (b) and (e). I choose (b) because from the formula $P = \frac{F}{A}$ then since the vessel A has more water, then the water will have a larger weight

Yes, vessel A has more water. The vessel is supporting more total weight of water. But the walls of vessel A are providing part of that support.

Think about an imaginary partition that separates the portion of the water above the sides from the portion of the water above the bottom. The water is at equilibrium either with or without the partition. Nothing is flowing anywhere. We can replace the imaginary partition with a rigid wall and nothing changes. But that just leaves us with a cylinder of water. Clearly the pressure on the bottom relates only to the depth of the water.

Just as Pascal's principle requires.

I choose (e) because I thought the pressure on the sides of A would be greatest since from each value of $h$ below the water, there is more water above in A since it circumference is largest at the top and decreases.

More water. More total force from the weight of that water. But some of that force is spread over the sloping sides above the given depth. Again, we could apply the partition argument and ignore both those sloping sides and the extra water above. Again, what does Pascal's principle say about the pressure of water at a given depth?

 

[kuruman]

It seems that someone did the experiment. Look at the pressure gauge readings.

 

[kuruman]

A related demonstration is the "connected vessels". If the pressure at the bottom of a vessel were different (say higher) than the others, fluid would flow out of it and lower its level. Also, as you can see, the cross sectional area at the bottom is irrelevant.

 

[Grelbr42]

Heh heh. Don't have that "desk toy" in places where certain smokables are still not legal.

 

[haruspex]

A related demonstration is the "connected vessels". If the pressure at the bottom of a vessel were different (say higher) than the others, fluid would flow out of it and lower its level. Also, as you can see, the cross sectional area at the bottom is irrelevant.

View attachment 326158

Note that if the levels were not equal one could construct a perpetual motion machine.

 

[member 731016]

Yes, vessel A has more water. The vessel is supporting more total weight of water. But the walls of vessel A are providing part of that support.

Think about an imaginary partition that separates the portion of the water above the sides from the portion of the water above the bottom. The water is at equilibrium either with or without the partition. Nothing is flowing anywhere. We can replace the imaginary partition with a rigid wall and nothing changes. But that just leaves us with a cylinder of water. Clearly the pressure on the bottom relates only to the depth of the water.

Just as Pascal's principle requires.

More water. More total force from the weight of that water. But some of that force is spread over the sloping sides above the given depth. Again, we could apply the partition argument and ignore both those sloping sides and the extra water above. Again, what does Pascal's principle say about the pressure of water at a given depth?

Thank you for your reply @jbriggs444 ! That is very helpful.

I assume that the water is at rest so we can use Pascal's principle. It states that change in pressure applied to a fluid at rest will be transferred though the fluid. Therefore, the increase in water will increase the weight, however, this therefore the upward sloping walls will increase their force to stop the water from falling though.

Do you know of a way to explain it better?

Many thanks!

 

[member 731016]

It seems that someone did the experiment. Look at the pressure gauge readings.

View attachment 326137

A related demonstration is the "connected vessels". If the pressure at the bottom of a vessel were different (say higher) than the others, fluid would flow out of it and lower its level. Also, as you can see, the cross sectional area at the bottom is irrelevant.

View attachment 326158

Note that if the levels were not equal one could construct a perpetual motion machine.

Thank you for your replies @kuruman and @haruspex!

 

[gmax137]

I think "d" (the force on the bottom of each vessel is not the same) is worth discussing. I would say the net force on the bottoms is the same: zero in every case (since the bottoms are not accelerating). Also, the water pressure at, and the area of, the bottoms are the same, so the force (P*A) on the bottom is the same. But the downward force at the "rims" of the bottoms - they're different aren't they? If you put these vessels on three scales, you'd see A is heavier than C, and C is heavier than B.

 

[jbriggs444]

Do you know of a way to explain it better?

When it comes to explanations, "better" tends to be personal. It is hard for any of us who are not professional teachers to know what sorts of explanations will resonate best with a particular student.

The easy explanation is: It's Pascal's principle. End of story.

If that does not resonate, then the imaginary partition idea works for me. Find a path from the surface to the point where you want to measure pressure. Put an imaginary partition around that path. Realize that making the partition solid and real will not change anything. Figure out the total pressure increment along that path and out pops the pressure at your chosen point.

That's pretty easy in this case -- just imagine a tower of fluid with a base that is one unit squared.

I had this basic idea in the back of my intuition for three or four years from the time I learned about buoyancy to the time when I finally took a course in multivarate calculus and learned about path integrals. I was mentally tiling the path with cubes and thinking about a limiting process until then. Pretty obvious stuff.

Then with multivarate calculus, you see that you have a simple conservative vector field with a potential that can be easily solved: $P = \rho g h$.