Probability flux integrated over all space is mean momentum?

[euphoricrhino]

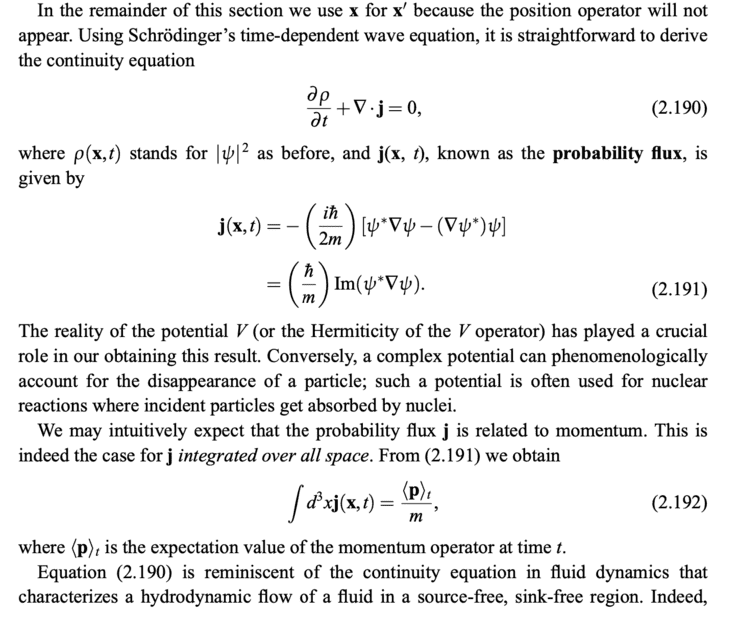
In Sakurai Modern Quantum Mechanics, I came across a statement which says probabiliy flux integrated over all space is just the mean momentum (eq 2.192 below). I was wondering if anybody can help me explain how this is obtained.
I can see that $i\hbar\nabla$ is taken as the $\mathbf{p}$ operator, but I don't see how the integration gives the mean of $\mathbf{p}$.
Thanks in advance!

 

[vanhees71]

The expectation value of the momentum is
$$\langle \vec{p} \rangle = \langle \psi|\hat{\vec{p}}|\psi \rangle = \int_{\mathbb{R}^3} \mathrm{d}^3 x \psi^*(t,\vec{x}) (-\mathrm{i} \hbar \vec{\nabla}) \psi(t,\vec{x}).$$
Now you can add the same expression with the $\nabla$ put to $\psi^*$ by partial integration and divide by 2:
$$\langle \vec{p} \rangle = \frac{1}{2} \int_{\mathbb{R}^3} \mathrm{d}^3 x (-\mathrm{i} \hbar) [\psi^*(t,\vec{x}) \vec{\nabla} \psi(t,\vec{x}) - \psi(t,\vec{x}) \vec{\nabla} \psi^*(t,\vec{x})].$$
Comparing this with Eq. (2.191) of the book you get immediately Eq. (2.192).

 

[euphoricrhino]

Great, thanks a lot.
I missed the integration by part trick. This is awesome!