Probability: posterior predictive probability

[Master1022]

Homework Statement

Suppose that the latest Jane arrived during the first 5 classes was 30 minutes. Find the posterior predictive probability that Jane will arrive less than 30 minutes late to the next class.

Relevant Equations

Probability

Hi,

This is a another question from the same MIT OCW problem in my last post. Nevertheless, I will try explain the previous parts such that the question makes sense. I know I am usually supposed to make an 'attempt', but I already have the method, but just don't understand it.

Questions:
1. Where has this posterior predictive probability come from (see image for part(c) solution)? It vaguely seems like a marginalization integral to me, but am confused otherwise.
2. Why are there two separate integrals for the posterior predictive probability over the different ranges of $x$ (see image for part(c) solution, but requires a result from part (b))? Would someone be able to explain that to me please?

Context:
Part (a)

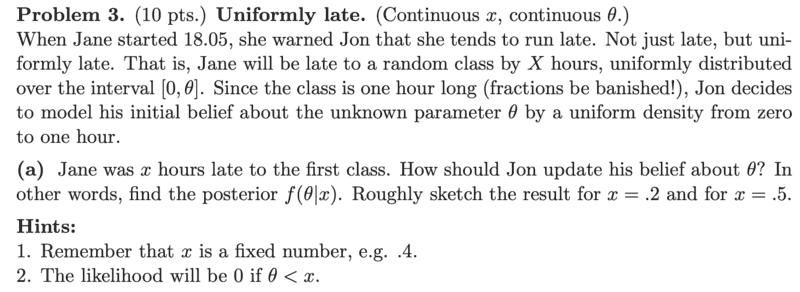
Part(a) solution:

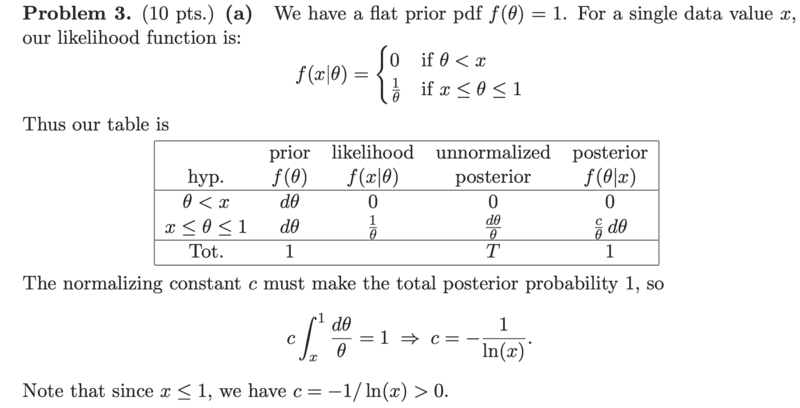Part (b):

Part (b) solution:

Part (c):

Part (c) solution: (this is what my question is about)

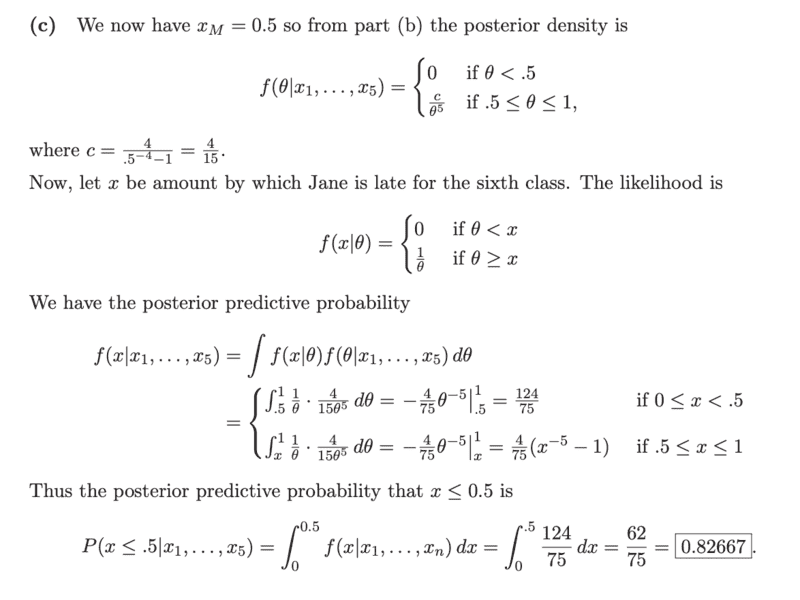Any help is greatly appreciated

 

[Orodruin]

1) Yes, it is marginalisation. You know the probability given $\theta$ and you know the probability of each $\theta$. The probability distribution for $x$ becomes the marginalised probability distribution. This is the continuous variable equivalent of $P(A|B) = P(A|C) P(C|B) + P(A|\bar C) P(\bar C | B)$ where $C$ and $\bar C$ are complementary.

2) There is one integral because you need to integrate the pdf for $x$ from 0 to 1/2. There is another integral arising from the fact that an integral appears in the marginalised probability.

 

[Master1022]

1) Yes, it is marginalisation. You know the probability given $\theta$ and you know the probability of each $\theta$. The probability distribution for $x$ becomes the marginalised probability distribution. This is the continuous variable equivalent of $P(A|B) = P(A|C) P(C|B) + P(A|\bar C) P(\bar C | B)$ where $C$ and $\bar C$ are complementary.

2) There is one integral because you need to integrate the pdf for $x$ from 0 to 1/2. There is another integral arising from the fact that an integral appears in the marginalised probability.

Thank you @Orodruin ! I will take some time to think about what you have written to internalize the content. However, just some initial follow up questions are:

With your answer to (2), I think that is starting make slightly more sense now. However, why has the solution provided an integral for the range $0.5 \leq x \leq 1$? It seems almost redundant to me...

 

[Orodruin]

With your answer to (2), I think that is starting make slightly more sense now. However, why has the solution provided an integral for the range 0.5≤x≤1? It seems almost redundant to me...

This is in the integral over $\theta$. While the observation makes $\theta > 1/2$ less likely, it is still a possibility that you need to take into account.

 

[Master1022]

This is in the integral over $\theta$. While the observation makes $\theta > 1/2$ less likely, it is still a possibility that you need to take into account.

Thanks for your reply. I'm really sorry to ask, but is there perhaps another way you can explain it as I am still struggling to understand it.

So what I understand is:
1. We have our posterior density function from part (b)
2. Now we want to predict the likelihood of Jane being less than 0.5 hours late to next one
3. We form the likelihood just as in part (a)
4. We need to consider all the different scenarios of $\theta$ and integrate over them

Why do we split up the range into $< 0.5$ and $0.5 \leq x \leq 1$? I know the 0.5 is what part of the main question.

Is it because the likelihood cannot be non-zero when $\theta < 0.5$. Therefore, $theta$ is limited by $min(x, 0.5)$ and 1? I am really sorry if this is worded poorly - I am finding it quite hard just to formulate exactly what I don't understand.

 

[Master1022]

Yesterday I realized that 'posterior predictive distributions' was another concept in itself so I went away to watch some videos on it. I didn't know about it before and was just coming from a background of knowing about MLE and MAP