Probability: Prior Distribution for a Continuous Variable

[Master1022]

Homework Statement

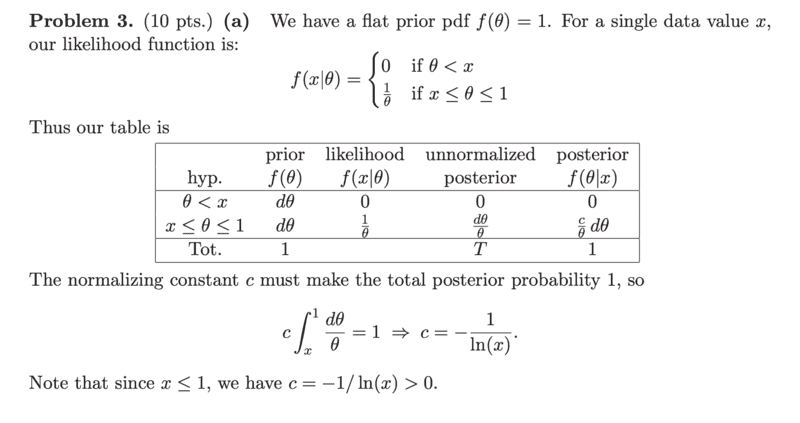
When Jane started class, she warned Jon that she tends to run late. Not just late, but uniformly late. That is, Jane will be late to a random class by $X$ hours, uniformly distributed over the interval $[0, \theta]$. Since the class is one hour long (fractions be banished!), Jon decides to model his initial belief about the unknown parameter $\theta$ by a uniform density from zero to one hour. (a) Jane was $x$ hours late to the first class. How should Jon update his belief about $\theta$? In other words, find the posterior $f(\theta|x)$. Roughly sketch the result for $x = .2$ and for $x = .5$.

Relevant Equations

Probability

Hi,

I was attempting this problem from the MIT OCW website probability and statistics course.

Context: When Jane started class, she warned Jon that she tends to run late. Not just late, but uniformly late. That is, Jane will be late to a random class by $X$ hours, uniformly distributed over the interval $[0, \theta]$. Since the class is one hour long (fractions be banished!), Jon decides to model his initial belief about the unknown parameter $\theta$ by a uniform density from zero to one hour. (a) Jane was $x$ hours late to the first class. How should Jon update his belief about $\theta$? In other words, find the posterior $f(\theta|x)$. Roughly sketch the result for $x = .2$ and for $x = .5$.

Quick Question: Why is the prior distribution $d\theta$? I cannot seem to understand that.

Picture from the solution:

Thanks

 

[lippyka]

!The prior distribution $d\theta$ is the probability density function (PDF) of the uniform distribution over the interval [0, θ]. It represents Jon's initial belief about the unknown parameter θ. The posterior $f(\theta|x)$ is the PDF of the updated belief about θ after observing that Jane was x hours late to the first class. The posterior can be calculated as:$f(\theta|x) = \frac{f(x|\theta)f(\theta)}{f(x)}$where $f(x|\theta)$ is the PDF of the uniform distribution from 0 to θ and $f(\theta)$ is the prior distribution. For example, if Jane was 0.2 hours late to the first class, then the posterior would be:$f(\theta|0.2) = \frac{\frac{1}{\theta} \frac{1}{1}}{\frac{1}{0.2}} = \frac{5}{\theta}$which has a peak at 0.2 and decreases monotonically as θ increases. If Jane was 0.5 hours late to the first class, then the posterior would be:$f(\theta|0.5) = \frac{\frac{1}{\theta} \frac{1}{1}}{\frac{1}{0.5}} = \frac{2}{\theta}$which has a peak at 0.5 and decreases monotonically as θ increases.