Probability to hit a spherical area

[LCSphysicist]

Homework Statement

.

Relevant Equations

.

I was asked to derive the relation $$p = u/3$$ for photon gas. Now, i have used classical mechanics and symmetry considerations, but the book has solved it in a interisting way:

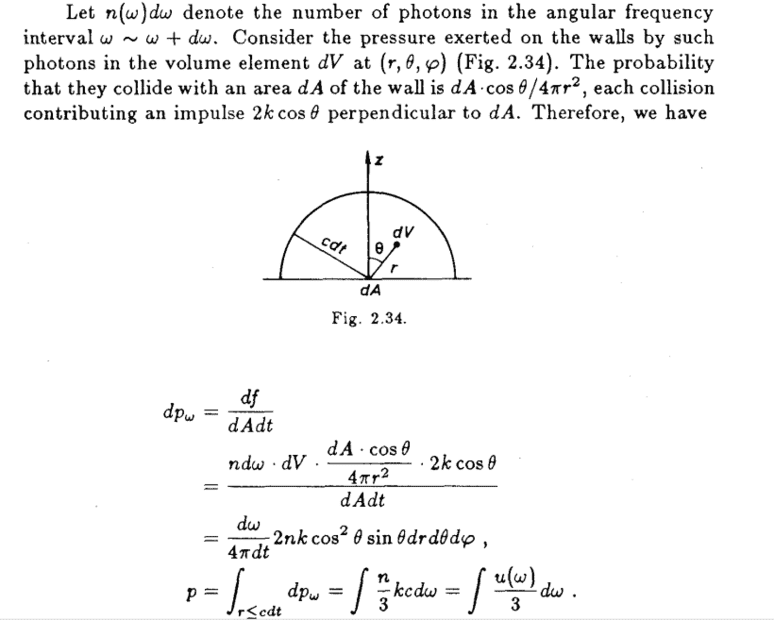
I can follow the whole solution given, the only problem is the one about the probability to colide the sphere!. Where does the $cos(\theta)$, in $dA cos(\theta)/(4 \pi r^2)$ comes from? Why the top part of the sphere is benefited?

 

[haruspex]

Where are these photons coming from?

 

[LCSphysicist]

Where are these photons coming from?

Damn i missed the "cavity" from the question. Please delete this topic :v

 

[haruspex]

Damn i missed the "cavity" from the question. Please delete this topic :v

Actually, there could be a flaw in the analysis. A photon that e.g. comes parallel to the z axis and strikes near the perimeter of the cavity will bounce around striking it in several other places.

 

[LCSphysicist]

Actually, there could be a flaw in the analysis. A photon that e.g. comes parallel to the z axis and strikes near the perimeter of the cavity will bounce around striking it in several other places.

Intresting. How could that be taken into account? If it were discrete pieces, i would guess a geometric series involving the probability. But not sure how someone would proceed here.

 

[haruspex]

Intresting. How could that be taken into account? If it were discrete pieces, i would guess a geometric series involving the probability. But not sure how someone would proceed here.

It certainly looks messy.
And I do not think it is the only problem. It treats the photons as arriving parallel to the z axis, whereas they would arrive equally likely from all directions at the base of the hemisphere, no? So it comes to whether the reflections are equally likely in all directions, or are favoured to be closer to or further from the z axis than their arrival paths.

In fact, why is this any different from an atomic gas?

Edit: I googled photon gas and found a treatment that assumes photons are absorbed and re-emitted, not reflected. But the algebra in post #1 seems to assume reflection.

 

[TSny]

Where does the $cos(\theta)$, in $dA cos(\theta)/(4 \pi r^2)$ comes from? Why the top part of the sphere is benefited?

The derivation considers photons reflecting off a small element of area $dA$ of the container wall during a small interval of time $dt$. For convenience, let $t = 0$ be the instant of time at the beginning of the interval $dt$. At $t = 0$ there is a certain number of photons within the container and some of these will strike $dA$ during the interval $dt$.

If a photon arrives at $dA$ at some instant within the interval $dt$, we can think of the photon as having been within some volume element $dV$ at the instant $t = 0$. In the figure, $dV$ represents one such volume element. It is evident that $dV$ must lie within a distance $cdt$ of $dA$ if any of its photons at $t = 0$ are to arrive at $dA$ during $dt$. The number of photons with frequencies between $\omega$ and $\omega + d\omega$ that are contained in $dV$ (at any instant of time, and particularly at $t = 0$) is $n(\omega) d\omega dV$. Only a certain fraction of these photons in $dV$ at $t = 0$ will be moving in a direction that will lead them to $dA$. Since we can assume an isotropic distribution of velocity directions of the photons, this fraction is the ratio of $dA \cos \theta$ to the total area of a sphere of radius $r$. Note that $dA \cos \theta$ is the projection of $dA$ perpendicular to $r$.

So, the number of photons that were in $dV$ at $t = 0$ that will strike $dA$ during $dt$ is $$n(\omega) d\omega dV \frac{ dA\cos\theta}{4 \pi r^2}$$

 

[haruspex]

The derivation considers photons reflecting off a small element of area dA of the container wall

Ah! I had not realized that line was a wall. I thought that we were looking at a hemispheric projection from the side of a container.