Astr
- 14
- 0
Hello, everyone.
This problem is easy if you assume that the friction between the blocks and the platform is the maximum possible. Then the normal force is 0. But how can you show that the normal is 0 even when the friction is less than maximum?
Thank you for your help.
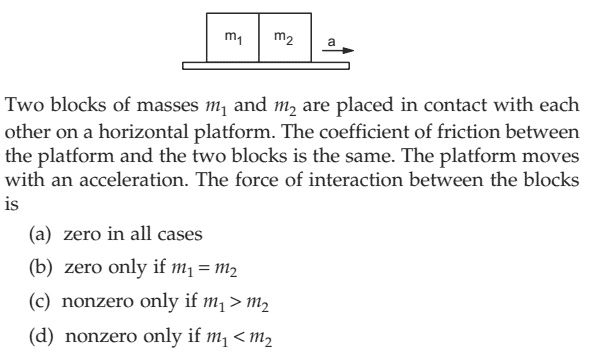
This problem is easy if you assume that the friction between the blocks and the platform is the maximum possible. Then the normal force is 0. But how can you show that the normal is 0 even when the friction is less than maximum?
Thank you for your help.