Problem with the sum of a Fourier series

[Amaelle]

Homework Statement

Look at the image

Relevant Equations

Fourier serie

Good day

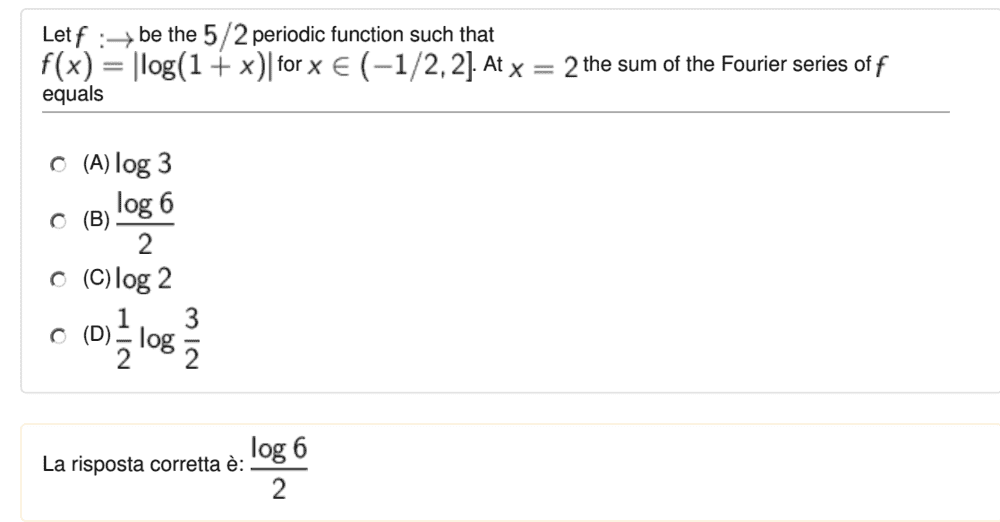
I really don't understand how they got this result? for me the sum of the Fourier serie of of f is equal to f(2)=log(3)
any help would be highly appreciated!
thanks in advance!

 

[BvU]

Hi,
Sounds reasonable. However:
what is the sum at $\ x=2+\epsilon\$ with $\ \ 0<\epsilon <<< 1\$ ?

 

[Amaelle]

thanks for your prompt answer,
as I saw it, I can't plug it the function f(x), so I really have no clue ...

 

[BvU]

The function is periodic, so $f(2+\epsilon) = f(-{1\over2}+\epsilon)$.
What is $f(-{1\over2})$ ?

 

[Amaelle]

f(-1/2)=f(2)=log(3) as they are periodic, right?

 

[BvU]

And it jumps down to what when you go a little further ?

 

[Amaelle]

so maybe I got you
In this region the value of f(-1/2)=0 so the we are in discountinous stepwise function, in which tthe value of f(2+e)
bounces between 2 and 0?

 

[Amaelle]

i mean jump between log3 and 0

 

[BvU]

$f(-{1\over2}) = 0$ ?

 

[pasmith]

$$\lim_{\epsilon \to 0}f(-\tfrac12 + \epsilon) = \lim_{\epsilon \to 0} |\log(-\tfrac12 + \epsilon)| = \lim_{\epsilon \to 0}\log(\tfrac{2}{1 - 2\epsilon}) = ?$$

 

[Amaelle]

$f(-{1\over2}) = 0$ ?

no I meant f(-1/2 +ε) =log(1+ε) =0 because -1/2 does not belong to the definition domain

 

[Amaelle]

$$\lim_{\epsilon \to 0}f(-\tfrac12 + \epsilon) = \lim_{\epsilon \to 0} |\log(-\tfrac12 + \epsilon)| = \lim_{\epsilon \to 0}\log(\tfrac{2}{1 - 2\epsilon}) = ?$$

log(2)

 

[Amaelle]

log(2)

but sorry just one question
lim f(-1/2 + epsilon)= lim |log(-1/2+epsilon)|=lim -log(-1/2+ epsilon)=lim log(2/(2epsilon-1)) I think there us a small problem with the sign no?

 

[BvU]

What is $\bigl |\log {1\over 2} \bigr |$ ?

 

[BvU]

because -1/2 does not belong to the definition domain

Correct, but does it make any difference for the Fourier series ? Do you calculate something different for $\ (-{1\over 2}, 2]\$ than for $\ [-{1\over 2}, 2)\$ ?

 

[Amaelle]

Correct, but does it make any difference for the Fourier series ? Do you calculate something different for $\ (-{1\over 2}, 2]\$ than for $\ [-{1\over 2}, 2)\$ ?

not does not

 

[Amaelle]

What is $\bigl |\log {1\over 2} \bigr |$ ?

|log(2)|=log(2)

 

[BvU]

Yes; I hope you got it right: $\ \bigl |\log{1\over 2}\bigr | = |-\log 2\bigr | = \log 2 \ .$

So at $x=2$ the Fourier sum jumps from $\log 3$ to $\log 2$. What's the average ?

$\$

 

[Amaelle]

log(6)/2, thanks a million!

 

[BvU]

So somehow the subtle difference between ranges $\ (-{1\over 2}, 2]\$ and $\ [-{1\over 2}, 2)\$ is 'lost in transformation'

$\$

 

[Amaelle]

So somehow the subtle difference between ranges $\ (-{1\over 2}, 2]\$ and $\ [-{1\over 2}, 2)\$ is 'lost in transformation'

$\$

yes , thanks a million, that was a nice explanation!