HRG
- 32
- 8
New trailer and new self built boat. Need confirmation of my calculation for placement of boat on trailer to have the correct tongue weight.
For those not familiar with trailers:
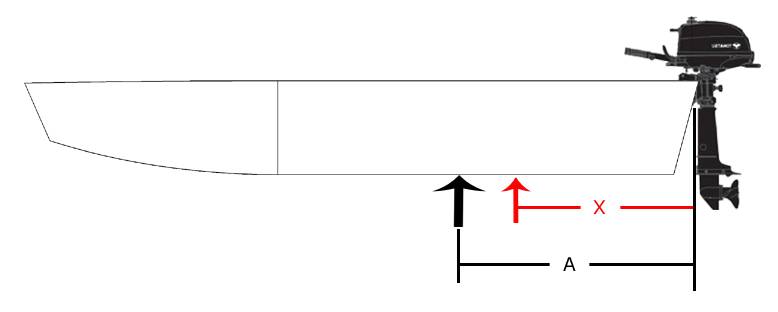
Assume that the total weight of the boat and motor is 250 lbs. When the boat is placed on the trailer, the total weight of trailer + boat will be 550 lbs (300lbs + 250lbs).
The black arrow points to the balance point of the boat with motor. If the boat is placed on the trailer with the black arrow over the wheel's axle, then the tongue weight will remain as 30 lbs which is wrong. The tongue weight at 10% of the total weight needs to be 55 lbs.
The red arrow points to where the boat should be placed over the axle to get 55 lbs of tongue weight.
My calculation is that X should be 80% of A to have the forward portion of the boat from the red arrow be 10% heavier than the aft portion of the boat from the red arrow. In other words, X=0.8A. Then if the boat is placed on the trailer with the red arrow above the axle, the tongue weight will be 10% of the total weight of the trailer + boat.
Is my calculation correct?
For those not familiar with trailers:
- The tongue of a trailer is the forward beam that has the female ball receiver at the front end.
- It is imperative that the weight of the trailer at the ball be 10-15 % of the total weight of the "trailer, boat, motor and anything in the boat". This results in steady, sway free pulling of the trailer by the vehicle at highway speeds.
- The commercially sold trailer with nothing on it will have the proper tongue weight for the empty trailer, to allow pulling the empty trailer safely. In other words, if the weight of the trailer is 300 lbs, then the tongue weight might be 10% or 30 lbs.
Assume that the total weight of the boat and motor is 250 lbs. When the boat is placed on the trailer, the total weight of trailer + boat will be 550 lbs (300lbs + 250lbs).
The black arrow points to the balance point of the boat with motor. If the boat is placed on the trailer with the black arrow over the wheel's axle, then the tongue weight will remain as 30 lbs which is wrong. The tongue weight at 10% of the total weight needs to be 55 lbs.
The red arrow points to where the boat should be placed over the axle to get 55 lbs of tongue weight.
My calculation is that X should be 80% of A to have the forward portion of the boat from the red arrow be 10% heavier than the aft portion of the boat from the red arrow. In other words, X=0.8A. Then if the boat is placed on the trailer with the red arrow above the axle, the tongue weight will be 10% of the total weight of the trailer + boat.
Is my calculation correct?