chwala
Gold Member
- 2,827
- 415
- Homework Statement
- Prove algebraically that ##n^3+3n-1## is odd for all positive integers ##n##.
- Relevant Equations
- Algebra
This is a past paper question; Find the solution here; Well understood
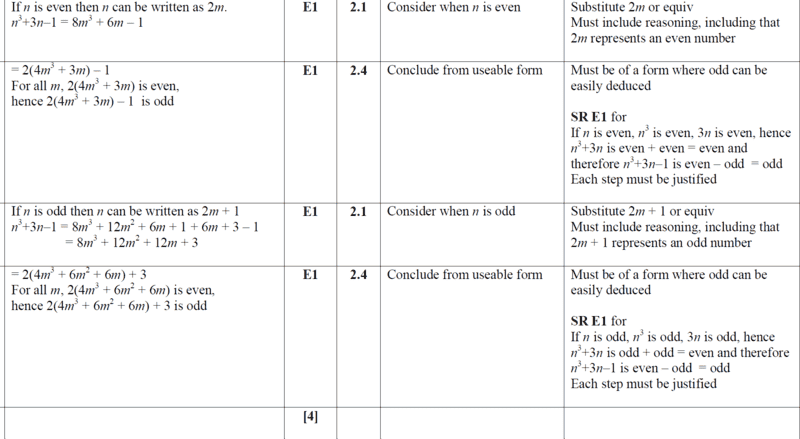
Now i was just thinking along this lines;
Let ##n=x##,
then ##f(x)=x^3+3x-1##
##f(x) =x(x^2+3)-1##
since ##x∈ℤ^{+}## then ##x(x^2+3)## will always be even implying that ##x(x^2+3)-1## is odd.
Would this approach hold or i have to stick with ms? Thanks.
Now i was just thinking along this lines;
Let ##n=x##,
then ##f(x)=x^3+3x-1##
##f(x) =x(x^2+3)-1##
since ##x∈ℤ^{+}## then ##x(x^2+3)## will always be even implying that ##x(x^2+3)-1## is odd.
Would this approach hold or i have to stick with ms? Thanks.