- #1
anemone
Gold Member
MHB
POTW Director
- 3,883
- 115
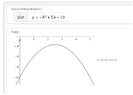
Prove that there is no root exists in the interval $(0,2)$ for a quartic function $k^4-10+k-3k^2$.