Prove Parallelogram Point P's Angles are Equal

[Albert1]

View attachment 972
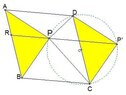
Point P is an iner point of a parallelogram ABCD
given $\angle PAB=\angle PCB$
please prove :$\angle PBA=\angle PDA$

 

[Albert1]

View attachment 986
Construct P'C//PB , and P'P//BC ,connecting P'D
now
BCP'P and APP'D are two parallelograms
it is easy to see
$\angle P'DC=\angle PAB=\angle PCB=\angle P'PC$
four points C,P'D,P are cyclic
$\therefore \angle DPP'=\angle DCP'$
but $\angle PDA=\angle DPP' , and\,\, \angle DCP'=\angle PBA \therefore \angle PBA=\angle PDA$