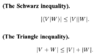Prove Schwarz Inequality for x, y, z in R+

[Pushoam]

Homework Statement

For x,y,z $\in \mathbb {R^+}$, prove that
$\sqrt {x (3 x +y) } + \sqrt {y (3y +z) } + \sqrt {z(3z +x)} \leq ~ 2(x +y+ z)$

Homework Equations

The Attempt at a Solution

I don't know which inequality among the above two has to be applied.
I am trying to solve it by inspection. I don't know the standard approach to solve it.

 

[mfb]

If in doubt, write the Schwarz inequality with components of general vectors v,w, expand the smaller side, then see if you can assign the components to get these square roots at a suitable spot in the equation.

 

[Pushoam]

If in doubt, write the Schwarz inequality with components of general vectors v,w, expand the smaller side, then see if you can assign the components to get these square roots at a suitable spot in the equation.

I did it. Thanks a lot for guiding me.