- #1
maxkor
- 84
- 0
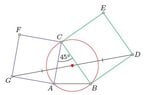
Let BCED and ACFG square. Prove that center of DG = center of the red circle.
View attachment 6025
I don't know how to start
View attachment 6025
I don't know how to start
Attachments
Last edited: