markosheehan
- 133
- 0
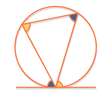
View attachment 6094
hi can someone help me work this out. i think it has something to do with the exterior angle of a triangle is equal to the sum of the interior angles but i can't work it.
hi can someone help me work this out. i think it has something to do with the exterior angle of a triangle is equal to the sum of the interior angles but i can't work it.