Proving convergence of rational sequence

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

The solution is,

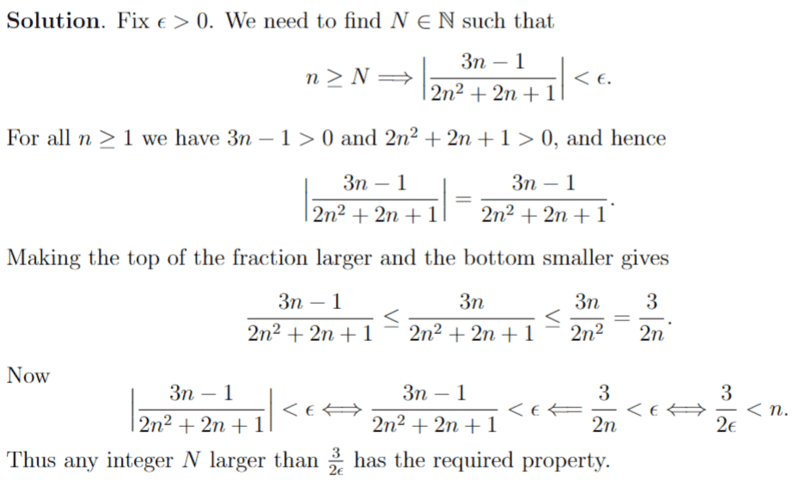
However, does someone please know why this did not use $2n ≤ 2n^2 + 2n + 1$ which would give

$\frac{3n - 1}{2n^2 + 2n + 1} ≤ \frac{3n}{2n} = \frac{3}{2}$?

In general, after solving many problems, it seems that when proving the convergence of a rational function from first principles, we want to find a expression for $\epsilon$ in terms of $n$ i.e $n(\epsilon)$, which is found by making sure that there is always a $n$ in the denominator so does not cancel when finding the fraction that bounds the sequence we are trying to prove convergence from i.e something of the form $\frac{a}{n^b}$ where $a$ is a constant and $b ≥ 1$ .

However, however, is $\frac{3}{2}$ allowed for proving that this rational function converges to zero? Please correct me if I am wrong, but it means that we know for sure that the rational function in this case is bounded above by $\frac{3}{2}$ but nothing else. To me, this seems anagolus to when you don't divide out polynomial solutions, but you solve for zero. Is that the same sort of case here?

I've never seen anybody talk about not eliminating the $n$ from first principles proofs of convergence in any real analysis textbook I have read.

Thanks for any help!

 

[Mark44]

In general, after solving many problems, it seems that when proving the convergence of a rational function from first principles, we want to find a expression for $\epsilon$ in terms of $n$ i.e $n(\epsilon)$, which is found by making sure that there is always a $n$ in the denominator so does not cancel when finding the fraction that bounds the sequence we are trying to prove convergence from i.e something of the form $\frac{a}{n^b}$ where $a$ is a constant and $b ≥ 1$ .

However, however, is $\frac{3}{2}$ allowed for proving that this rational function converges to zero? Please correct me if I am wrong, but it means that we know for sure that the rational function in this case is bounded above by $\frac{3}{2}$ but nothing else. To me, this seems anagolus to when you don't divide out polynomial solutions, but you solve for zero. Is that the same sort of case here?

When you a limit of a sequence using the definition of the limit, you need to determine the number n that depends on the given $\epsilon$. [/quote]

In the work that you did, you established that $\frac{3n - 1}{2n^2 + 2n + 1} ≤ \frac{3n}{2n} = \frac{3}{2}$, but that's not what you were asked to do. For large n, $\frac{3n - 1}{2n^2 + 2n + 1} ≤\frac{3}{2}$, but what you're asked to do is to show that the rational expression can be made smaller than any given positive number $\epsilon$. IOW, that the limit of this expression is 0.

 

[nuuskur]

Alternatively,
$$\frac{3n-1}{2n^2+2n+1} = \frac{3-\frac{1}{n}}{2n+2+\frac{1}{n}} < \varepsilon \Leftrightarrow \frac{2n+2+\frac{1}{n}}{3-\frac{1}{n}} > \frac{1}{\varepsilon} \Leftarrow 2n+2+\frac{1}{n}> \frac{3}{\varepsilon} \Leftarrow 2n > \frac{3}{\varepsilon},$$
which implies taking $n > \frac{3}{2\varepsilon}$ is sufficient. There are many paths to victory. The end goal is to find an index such that all indices after make the expression under the limit sign smaller than $\varepsilon$. Once we have $N> \frac{3}{2\varepsilon}$ we could also take $N> \frac{3}{2\varepsilon} + 10^6$ or what have you.