Proving function discontinuous at zero

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

THe solution is,

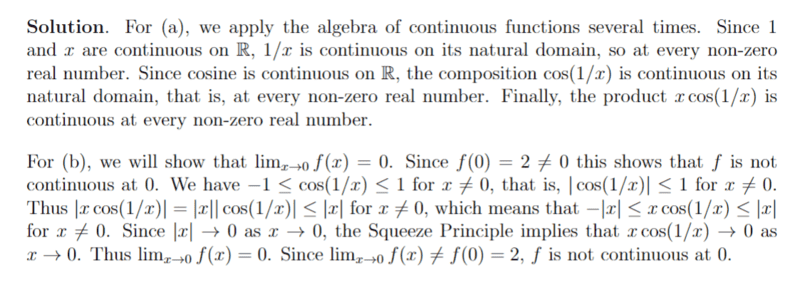
However, does someone please know why from this step $-1 \leq \cos(\frac{1}{x}) \leq 1$ they don't just do $-x \leq x\cos(\frac{1}{x}) \leq x$ from multiplying both sides by the monomial linear function $x$

$\lim_{x \to 0} - x = \lim_{x \to 0} x= 0$ then use squeeze principle and reach the same conclusion as them

Thanks!

 

[FactChecker]

When $x$ goes to 0, you do not know if it is positive or negative. If it is negative, then $-x \le x$ is false. Using the absolute values, $-|x| \le |x|$ solves that problem.

 

[fresh_42]

If $f(x)$ is a continuous function, then $\lim_{x \to a} f(x)= f(a)\;\;(*).$ Hence, if we assume - in order to derive a contradiction - that $f(x)=x \cdot \cos(x^{-1})$ was continuous, and $-|x|\leq f(x)\leq |x|$ for $x\neq 0$, then both together results in
$$
0=\lim_{x \to 0} (-|x|) \leq \lim_{ \array{ x \to 0 \\ x\neq 0} } f(x)=\lim_{ \array{ x \to 0 \\ x\neq 0} }(x\cdot\cos(x^{-1}))\leq \lim_{x \to 0}|x|=0.
$$
With $(*)$ we get $\displaystyle{\lim_{\array{ x \to 0 \\ x\neq 0}} f(x)=\lim_{\array{ x \to 0 \\ x\neq 0}}(x\cdot\cos(x^{-1}))=f(0)=0}$ if $f(x)$ was continuous. Since $f(0)=2,$ $f(x)$ cannot be continuous at $x=0.$