Proving rational function converges from first principles

[member 731016]

Homework Statement

Please see below

Relevant Equations

Epsilon-Delta definition of a limit

For this problem,

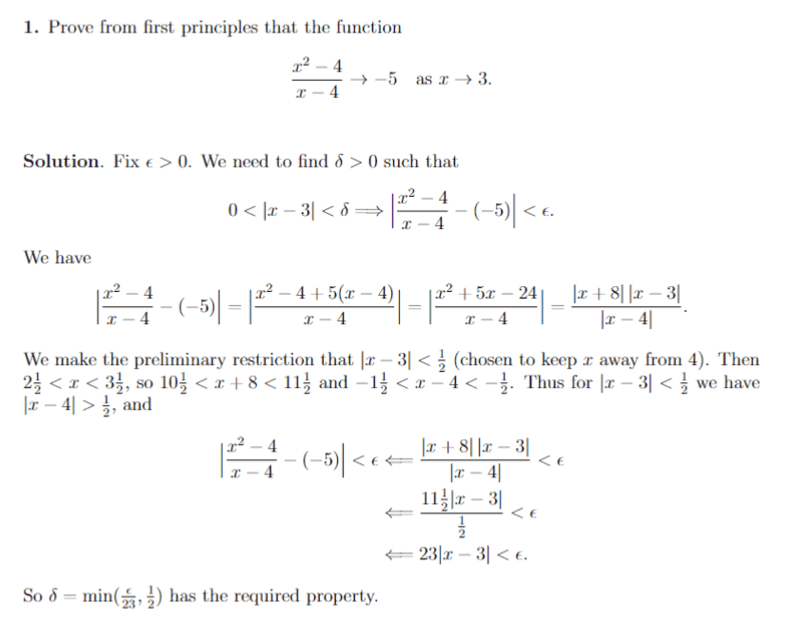
I am confused how they get $$| x - 4 | > \frac{1}{2}$$ from. I have tried deriving that expression from two different methods. Here is the first method:

$$-1\frac{1}{2} < x - 4 < -\frac{1}{2}$$
$$1\frac{1}{2} > -(x - 4) > \frac{1}{2}$$
$$|1\frac{1}{2}| > |-(x - 4)| > |\frac{1}{2}|$$
$$1\frac{1}{2} > |-1||x - 4| > \frac{1}{2}$$
$$1\frac{1}{2} > |x - 4| > \frac{1}{2}$$
Thus $$ |x - 4| > \frac{1}{2}$$

However, I also have an alternative method, but I am unsure why it is not giving the correct expression. Here the second method:

$$-1\frac{1}{2} < x - 4 < -\frac{1}{2}$$
$$|-1\frac{1}{2}| < |x - 4| < |-\frac{1}{2}|$$
$$1\frac{1}{2} < |x - 4| < \frac{1}{2}$$

Does someone please know what I have done wrong?

Thank you - Chiral

 

[FactChecker]

I am confused how they get $$| x - 4 | > \frac{1}{2}$$ from.

That is because $|x-3|<\frac {1}{2}$ so $x$ is closer to 3 than it is to 4..

 

[member 731016]

That is because $|x-3|<\frac {1}{2}$ so $x$ is closer to 3 than it is to 4..

Thank you for your reply @FactChecker!

Sorry I am still confused. I am trying to understand how they algebraically derive $$|x - 4| > \frac{1}{2}$$

Thanks!

 

[FactChecker]

1) You can look on the number line and see it geometrically.
or
2) |x-3| <1/2
=> x-3 < 1/2
=> x-4 < 1/2-1 = -1/2
=> 4-x > 1/2
=> |x-4| > 1/2

 

[member 731016]

1) You can look on the number line and see it geometrically.
or
2) |x-3| <1/2
=> x-3 < 1/2
=> x-4 < 1/2-1 = -1/2
=> 4-x > 1/2
=> |x-4| > 1/2

Thank for your reply @FactChecker!

However, I am still confused. Do you please know why you only consider the positive case when you take off the absolute value off x - 3 i.e not $$-(x - 3) < 1/2$$?

Do you also please know whether my two algebraic methods are correct?

Thanks!

 

[FactChecker]

$$-1\frac{1}{2} < x - 4 < -\frac{1}{2}$$
$$|-1\frac{1}{2}| < |x - 4| < |-\frac{1}{2}|$$

This is your error. $x-4 \lt -\frac{1}{2}$ does not imply $|x-4|\lt |-\frac{1}{2}|$.

 

[member 731016]

This is your error. $x-4 \lt -\frac{1}{2}$ does not imply $|x-4|\lt |-\frac{1}{2}|$.

Thank you for your reply @FactChecker!

That is interesting, do you please know why? I took the absolute value of all three sides of the equation from the law of algebra. I thought they would be equivalent since I did the same thing to each side of the equation.

Thanks!

 

[FactChecker]

That is interesting, do you please know why? I took the absolute value of all three sides of the equation from the law of algebra. I thought they would be equivalent since I did the same thing to each side of the equation.

These are not equations. When you have a comparison like $-100 \lt 1$, which is not an equation, you can not just do the same thing to both sides and know that the same comparison still works.
$-100 \lt 1$ does not mean that $|-100 |\lt |1|$.

 

[FactChecker]

It there is equality, then any function applied to equal things will give equal answers. If $x1=x2$, then $f(x1)=f(x2)$. Otherwise, the function $f()$ is not well defined. But if there is some other relationship between $x1$ and $x2$ different from equality, that can not be guarantied.