Proving SHM for charged spring mass system in electric field

AI Thread Summary
The discussion focuses on understanding the derivative of a constant in the context of a charged spring-mass system in an electric field. The user initially questions why a specific highlighted statement is true, particularly regarding the constant value x_0. They realize that the derivative of x_0 with respect to time is zero, indicating no change in the rest position over time. This rest position is defined by the balance of forces acting on the block. The user expresses gratitude for the clarification received.
Nway
Messages
34
Reaction score
0
Homework Statement
Problem below.
Relevant Equations
Problem below.
For part (f)
1674159276690.png

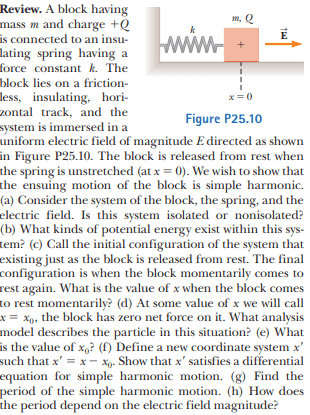
Solution is
1674159332251.png


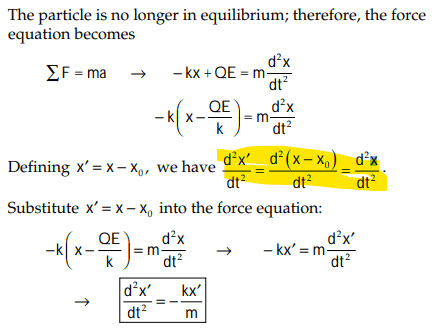
I don't understand why the bit highlighted in yellow is true.

Would anybody be kind enough to help.
 
Physics news on Phys.org
x_0 is a constant value. What is the derivative of constants?
 
nasu said:
x_0 is a constant value. What is the derivative of constants?
I see now. ## \frac {dx_0} {dt} = 0 ## as for each differential time, there is no change in the rest position. This is because rest position is function of where the forces acting on the block are equal only. Right?

Thank you for the help @nasu .
 

Thread 'Rolling without slipping on a curved surface'

Kindly see the attached pdf. My attempt to solve it, is in it. I'm wondering if my solution is right. My idea is this: At any point of time, the ball may be assumed to be at an incline which is at an angle of θ(kindly see both the pics in the pdf file). The value of θ will continuously change and so will the value of friction. I'm not able to figure out, why my solution is wrong, if it is wrong .
View full post »
Thread 'Voltmeter readings for this circuit with switches'

Thread 'Voltmeter readings for this circuit with switches'

TL;DR Summary: I would like to know the voltmeter readings on the two resistors separately in the picture in the following cases , When one of the keys is closed When both of them are opened (Knowing that the battery has negligible internal resistance) My thoughts for the first case , one of them must be 12 volt while the other is 0 The second case we'll I think both voltmeter readings should be 12 volt since they are both parallel to the battery and they involve the key within what the...
View full post »
Thread 'Trying to understand the logic behind adding vectors with an angle between them'

Thread 'Trying to understand the logic behind adding vectors with an angle between them'

My initial calculation was to subtract V1 from V2 to show that from the perspective of the second aircraft the first one is -300km/h. So i checked with ChatGPT and it said I cant just subtract them because I have an angle between them. So I dont understand the reasoning of it. Like why should a velocity be dependent on an angle? I was thinking about how it would look like if the planes where parallel to each other, and then how it look like if one is turning away and I dont see it. Since...
View full post »

Similar threads

Electric field of point along the central vertical axis of a triangle
Replies
3
Views
878
Spring-mediated dipole in a magnetic field
Replies
31
Views
2K
Amplitude of a mass joined to a spring in the presence of an E-field
Replies
5
Views
2K
Symmetry behind charged spring-mass system in Electric field
Replies
2
Views
1K
Distribution of Energy when work is done on a system of 2 masses connected by a spring
Replies
17
Views
2K
Does amplitude depend on mass in SHM?
Replies
1
Views
8K
Stretching of a rotating spring
Replies
8
Views
2K
Spring momentum conservation problem
Replies
4
Views
2K
The electric potential inside a conducting sphere with charge Q
Replies
11
Views
2K
Find spring constant k using an electrical circuit
Replies
10
Views
2K

Hot Threads

Recent Insights

Back
Top