Proving the Infimum and Supremum: A Short Guide for Scientists

[Lambda96]

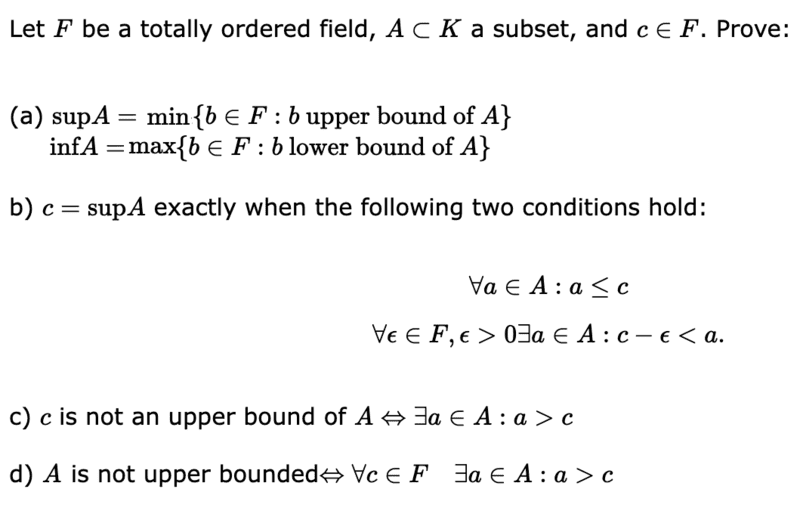
Homework Statement

proof that b is the supremum of supA
proof that b is the Infimum of infA

Relevant Equations

none

Hi,

I have problems with the proof for task a

I started with the supremum first, but the proof for the infimum would go the same way. I used an epsilon neighborhood for the proof

I then argued as follows that for $b- \epsilon$ the following holds $b- \epsilon < b$ and $b- \epsilon \in A$ for $b+ \epsilon$ then $b+ \epsilon > b$ and thereby $b+ \epsilon \notin A$ holds.

By the fact that I can make the epsilon arbitrarily small and thereby the above properties still hold, b must be the smallest upper bound of A.

Would this be sufficient as a proof?

 

[FactChecker]

I then argued as follows that for $b- \epsilon$ the following holds $b- \epsilon < b$ and $b- \epsilon \in A$ for $b+ \epsilon$ then $b+ \epsilon > b$ and thereby $b+ \epsilon \notin A$ holds.

Break this up into several sentences that are more clear and carefully stated. You say that $b - \epsilon$ is in $A$ and not in $A$. That can not be true.

 

[WWGD]

It seems you must have been given a definition of the sup, inf , in order to do the proof.

 

[Lambda96]

Thanks for your help FactChecker and WWGD, in the script from my professor it says the following.

$\textbf{supremum}$
An element $c \in F$ is called least upper bound or supremum of A, denoted by $\text{sup}$A, if the following properties are satisfied.

i) $a \le c$ for all $a \in A$.
ii) If b is an upper bound of A, then $c \le b$ follows.$\textbf{infimum}$
An element $c \in F$ is called greatest lower bound or infimum of A, denoted by $\text{inf}$A, if the following properties are satisfied:

i)$a \ge c$ for all $a \in A$.
ii)If b is a lower bound of A, then $c \ge b$ follows.