Proving trigonometry identities

[chwala]

Homework Statement

see attached

Relevant Equations

trigonometry concept

I was just looking at the problem below: there may be several ways to prove the identity:

question:

Mark scheme solution:

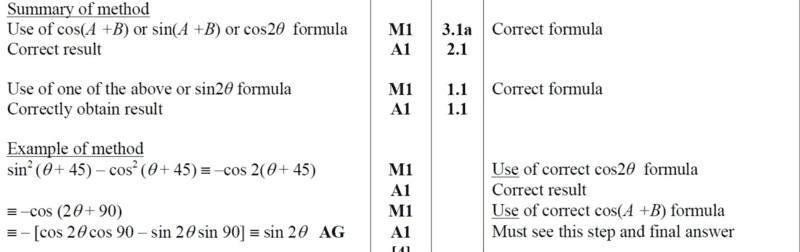
My take:
we may also use $sin^{2}x+cos^{2}x≡(sin x+ cos x)(sin x-cosx)$...
we end up with($\frac 2 {\sqrt{2}}$cos ∅)($\frac 2 {\sqrt{2}}$sin ∅)=$2 sin ∅ cos ∅$= $sin 2∅$

 

[anuttarasammyak]

The formula
cos(\alpha+\beta)=\cos\alpha cos\beta - \sin \alpha \sin \beta
is helpful.

 

[chwala]

The formula
cos(\alpha+\beta)=\cos\alpha cos\beta - \sin \alpha \sin \beta
is helpful.

True, that's what I used...together with the one for sine...

 

[Mark44]

My take:
we may also use $sin^{2}x+cos^{2}x≡(sin x+ cos x)(sin x-cosx)$...

No, that's incorrect.
$\sin^2(x) + \cos^2(x) = 1$, for all x.
If you meant $\sin^2(x) - \cos^2{x}$, that that does factor as you show above.

we end up with($\frac 2 {\sqrt{2}}$cos ∅)($\frac 2 {\sqrt{2}}$sin ∅)=$2 sin ∅ cos ∅$= $sin 2∅$

 

[chwala]

No, that's incorrect.
$\sin^2(x) + \cos^2(x) = 1$, for all x.
If you meant $\sin^2(x) - \cos^2{x}$, that that does factor as you show above.

yeah, true...i made a slight mistake there...it should be the difference of two squares, thanks mark...i.e
$[sin(∅+45)+cos(∅+45)]⋅[sin(∅+45)-cos(∅+45)]$
$[(sin ∅cos 45+cos ∅sin 45)+(cos ∅cos 45-sin ∅sin 45)]⋅ [(sin ∅cos 45+cos ∅sin 45)-(cos ∅cos 45-sin ∅sin 45)]$
thanks Mark cheers.